Adjacent Angles – Definition With Examples
Updated on January 8, 2024
Adjacent angles are a concept that resonates with the world around us, from the architecture of the buildings we inhabit to the art we admire. At Brighterly, we believe in taking abstract mathematical principles and making them engaging and comprehensible. It’s with this vision that we delve into adjacent angles, one of the essential building blocks of geometry. Whether you are a curious learner, a concerned parent, or an enthusiastic educator, this exploration of adjacent angles is designed to equip you with the understanding and appreciation of this foundational mathematical concept. Together, we will unlock the mysteries of adjacent angles, delve into their properties, explore the differences, and learn how to make calculations and solve problems that revolve around them.
What Are Adjacent Angles?
Angles are integral to understanding geometry, but what about the specific category called adjacent angles? In mathematics, angles are everywhere! Whether it’s the corner of a square or the twist in a spiral staircase, angles make up the world around us. Let’s dive into adjacent angles and see what makes them so interesting!
Definition of Angles
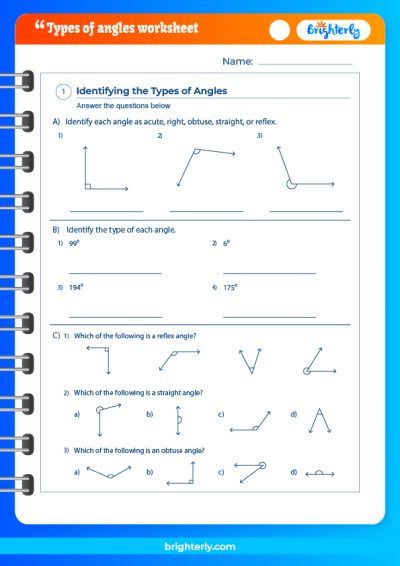
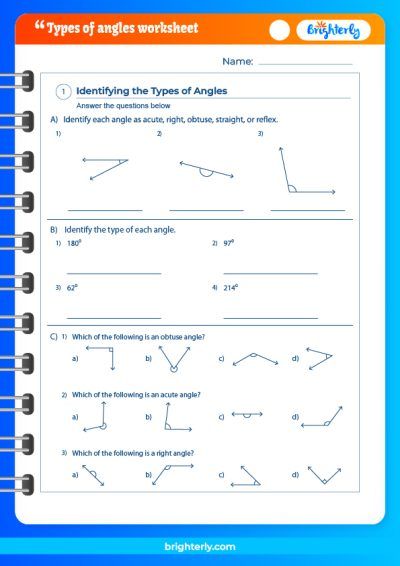
Angles are formed when two rays meet at a common endpoint, known as a vertex. Imagine opening a book and considering one page as one ray and the other as the second ray. The angle measures the amount of turn between the two rays, and it’s measured in degrees. Angles can range from 0° all the way up to 360°. They are often categorized into three types: acute (less than 90°), obtuse (more than 90° but less than 180°), and right angles (exactly 90°).
Definition of Adjacent Angles
Adjacent angles are a pair of angles that share a common vertex and a common arm, but do not have any common interior points. Think of them as neighbors; they live side by side without overlapping. If you have two angles, ∠A and ∠B, and they share a common side and vertex, they are adjacent angles. This concept is essential in geometry, especially in polygon shapes.
Properties of Angles
Angles are full of intriguing properties! From being categorized into complementary (adding up to 90°), supplementary (adding up to 180°), or vertical (opposite angles formed by intersecting lines), angles are abundant in variety. An angle’s size doesn’t change even if you rotate or move it. In other words, if two angles have the same measure, they are congruent. These properties form the foundation of geometry.
Properties of Adjacent Angles
The properties of adjacent angles are even more fascinating. Since adjacent angles share a common side, the rays that form these angles lie next to each other. If the adjacent angles are supplementary, they can form a straight line. Adjacent angles often appear in parallel lines cut by a transversal, a fundamental concept in Euclidean geometry.
Difference Between Angles and Adjacent Angles
While all adjacent angles are angles, not all angles are adjacent! Adjacent angles must meet the specific condition of sharing a common vertex and a common arm. In contrast, angles, in general, may or may not meet these criteria. Understanding this difference is vital in mathematical reasoning and applications.
Calculations Involving Adjacent Angles
Calculating adjacent angles is a key skill in geometry. If you know the measure of one adjacent angle and the two angles are supplementary, you can find the other angle by subtracting from 180°. These calculations often form the basis for solving more complex geometric problems.
Writing Equations Involving Adjacent Angles
Adjacent angles can lead to intriguing algebraic equations. For example, if two adjacent angles are supplementary and one angle is twice the other, you can write an equation like x + 2x = 180, where x is the measure of the smaller angle. Equations like these build the bridge between algebra and geometry.
Solving Problems Involving Adjacent Angles
Solving problems with adjacent angles involves applying the principles and calculations previously discussed. It may require utilizing complementary or supplementary angle properties, writing equations, or geometric visualization. The process is often intricate and requires a clear understanding of mathematical concepts.
At Brighterly, we believe that practice is the key to mastery. That’s why we invite you to explore our adjacent angles worksheets, where you can find an array of additional practice questions, complete with answers.
Practice Problems on Adjacent Angles
- If
∠Aand∠Bare adjacent angles, and∠A = 60°, what is the measure of∠Bif they are supplementary? - Write an equation representing two adjacent angles where one is three times the other, and they add up to form a straight line.
Conclusion
As we’ve journeyed through the concept of adjacent angles, we’ve not only encountered the mathematical principles but the real-world applications that bring these angles to life. At Brighterly, we believe that knowledge should be accessible, engaging, and empowering. Understanding adjacent angles is not merely an academic endeavor; it’s about connecting with the very framework of the world around us. From recognizing the geometry in nature’s design to applying this knowledge in various fields like engineering, art, and design, adjacent angles are a vital part of our daily life. With this comprehensive guide, we hope to have illuminated the subject in a way that inspires curiosity and encourages further exploration. After all, mathematics is not just about numbers; it’s about patterns, relationships, and understanding the world in a whole new way.
Frequently Asked Questions on Adjacent Angles
What are the applications of adjacent angles?
Adjacent angles are present everywhere, from the design of bridges to the creation of beautiful artworks. Engineers at companies like Brighterly often use adjacent angles to create precise structures. Artists might use them to generate symmetry in their works. These angles are also integral in computer graphics, navigation systems, and even in understanding the movement of celestial bodies. Truly, adjacent angles are not confined to textbooks; they breathe life into our world.
How do I teach adjacent angles to children?
Teaching adjacent angles to children can be a joyful experience. At Brighterly, we recommend a hands-on approach using visual aids such as drawings, models, and interactive digital tools. Start by explaining the concept using simple language and everyday examples like the hands of a clock or the angles in a book. Engage children in activities like drawing or constructing angles, using interactive geometry software, or even exploring angles in nature. Making the learning process fun and relatable helps children grasp the concept with ease and interest.
Can adjacent angles be equal?
Yes, adjacent angles can be equal, and this is a concept that we often explore in the Brighterly curriculum. For example, in a parallelogram, opposite sides are parallel, and adjacent angles are equal. When two lines are intersected by a transversal in such a way that corresponding angles are equal, adjacent angles between those lines will also be equal. Understanding this property of adjacent angles can lead to deeper insights into geometry and enrich a child’s mathematical thinking.
Information Sources
Online Winter Math Camp for Grades 1-8
Join us to help your kid achieve their full math potential.
Choose kid's grade
Online Winter Math Camp for Grades 1-8
Join our Math Winter Camp and boost your child's math skills with our best tutors.
