Angle Measure
Updated on January 1, 2024
Greetings, young explorers! Welcome aboard the Brighterly express, the place where learning is an adventure. We are on a mission to bring light to the sometimes mysterious world of math, making it as fun and exciting as a treasure hunt. Today, we’re setting sail to the intriguing island of “angle measure”.
Angles are everywhere! From the corners of a book to the hands on a clock, angles are an integral part of our lives. They help architects design buildings, pilots navigate flights, and even game developers create realistic scenes. That’s why understanding angles is like learning a secret language that can help you decode the world around you.
At Brighterly, we believe that every child has the potential to shine brightly in the world of mathematics. We’ve designed this guide to make learning about angles as enjoyable as playing your favorite game. So, buckle up, bring along your curiosity, and let’s dive into the fascinating world of angle measure together!
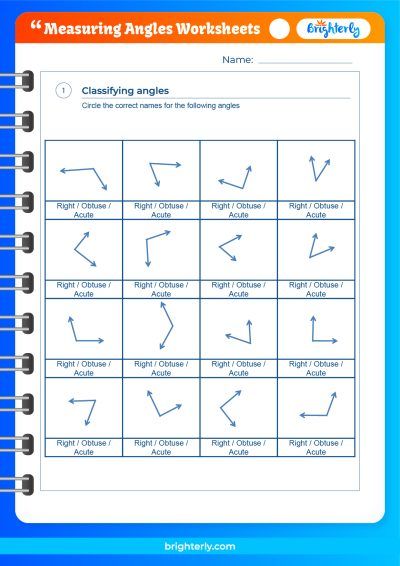
Types of Angles
In the vibrant world of geometry, angles are the colorful characters that bring the story to life. They each have a unique role to play and are defined by their distinctive characteristics. Just as we appreciate the diversity of personalities in our friends, we can also celebrate the variety of angles in mathematics. Let’s meet these interesting characters now!
Types of Angles Based on Measurement
Right Angle
Meet the celebrity of the geometry world—the Right Angle! It always measures exactly 90 degrees, striking a pose that’s as familiar to us as the corners of a square or a rectangle. You’ll find it everywhere, from the corners of a book to the intersections of city streets. The right angle is not just right—it’s essential!
Acute Angle
Next, we have the darling of the angle family—the Acute Angle. This cute little angle measures less than 90 degrees, making it smaller than a right angle. However, don’t let its size fool you! From the sharp tips of a star to the edges of a diamond, acute angles create the delicate details that make geometric shapes fascinating.
Obtuse Angle
Here comes the Obtuse Angle, a gentle giant in the world of angles. Measuring between 90 and 180 degrees, it’s larger than a right angle but less than a straight angle. It gives a sense of openness and expansion, creating wider perspectives in geometric shapes.
Straight Angle
Say hello to the balanced and steady Straight Angle. This angle is a half-turn, a straight line, measuring 180 degrees. It’s the baseline, the leveler, the path that takes us from one point to another in the most direct way. In geometry, the straight angle is our constant guide, leading us straight to our destination.
Reflex Angle
Last but not least, we have the Reflex Angle, the adventurer of angles. Measuring between 180 and 360 degrees, it’s more than a half-turn but less than a full turn. It offers an unconventional point of view, challenging us to see beyond the ordinary and explore the world of geometry from a different angle.
Just as every friend in your group brings something unique to the table, each of these angles has its own special role in the world of geometry. They come together to create a wide range of shapes and structures, adding depth and dimension to our world. Understanding these different types of angles is a stepping stone towards becoming a master geometrist with Brighterly!
Unveil the captivating world of angle measure with Brighterly Math for Children. From understanding different types of angles to mastering angle pairs, our interactive and engaging content makes learning angles a magical experience. With solved examples and practice problems, young learners can build confidence in measuring angles. Join us on this exciting journey and empower your child’s mathematical growth with Brighterly!
Types of Angles Based on Rotation
Just like how a compass guides an adventurer, understanding the concept of rotation in angles can guide us through the labyrinth of geometry. Let’s delve into the world of Positive Angles and Negative Angles.
Positive Angles
Positive Angles are the rebel angles. They like to go against the flow and are measured in the counterclockwise direction. Imagine the hands of a clock moving backward, defying time—that’s how positive angles roll!
Negative Angles
On the flip side, we have the Negative Angles. They’re traditionalists, adhering to the clockwise direction, similar to the normal movement of a clock’s hands. They follow the flow and help us keep track of time.
Types of Angle Pairs
Just like how characters in a story form relationships, angles too can pair up and share special bonds. These pairs, with their unique relationships, add another layer of intrigue to the captivating world of geometry.
Adjacent Angles
Adjacent Angles are the best buddies of the angle world. They share a common vertex and a common side, but don’t have any common interior points. They sit side by side, like two friends sharing secrets on a park bench.
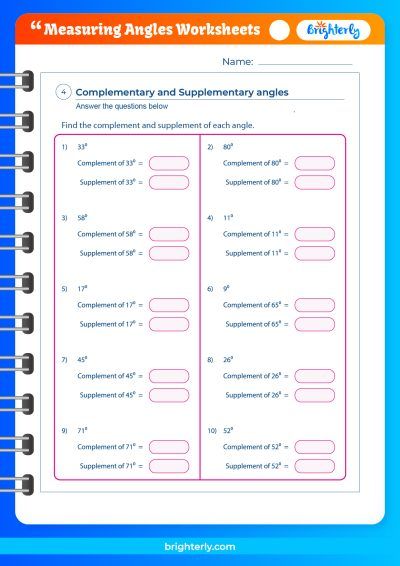
Complementary Angles
Complementary Angles, as their name suggests, complement each other perfectly. These angles are so in sync that their measures add up to 90 degrees. Just like two pieces of a puzzle fitting perfectly together, they complete each other to form a right angle.
Supplementary Angles
Supplementary Angles are the partners in crime that always have each other’s back. These angles work together so well that their measures add up to 180 degrees. When they come together, they create a straight angle, just like a magic trick!
Corresponding Angles
Corresponding Angles are the synchronized swimmers of the angle world. They maintain the same relative position in two different geometric shapes or when two parallel lines are intersected by a transversal. These angles are mirror images of each other, creating a beautiful symmetry in geometric shapes.
Vertical Angles
Lastly, we have the Vertical Angles, the twins of the angle world. They are formed by the intersection of two lines and are always opposite each other. What’s fascinating about these angles is that they always have equal measures, like two peas in a pod!
Solved Examples on Angle Measure
Unleash the detective in you with our solved examples on angle measure. By working through these examples, you’ll see these concepts in action and learn how to apply them in various problems. Get ready to solve some mathematical mysteries!
Practice Problems on Angle Measure
Ready for a challenge? It’s time to put your knowledge to the test with our practice problems on angle measure. These problems will help you gain confidence in your angle-measuring skills, preparing you to tackle any angle-related problem that comes your way. Remember, practice makes perfect, and at Brighterly, we’re all about helping you shine brighter!
Conclusion
Congratulations, intrepid learners! You’ve successfully navigated through the wide world of angle measure. As part of the Brighterly family, you’ve embraced the challenge and ventured into a significant area of mathematics, coming out the other side brimming with new knowledge.
Understanding angle measure isn’t just a stepping stone towards mastering geometry—it’s a gateway to seeing the world from a new perspective. From the rising and setting of the sun to the construction of magnificent skyscrapers, angles play a fundamental role in our daily lives.
But remember, every great explorer knows that the journey of learning never ends. There’s always more to discover and understand. Keep questioning, keep exploring, and continue to grow your love for learning. With Brighterly, you’re not just learning math—you’re embarking on an adventure, developing skills that will empower you to shine brightly in any path you choose.
So, keep practicing, keep shining, and remember—math isn’t something to be feared. It’s a fascinating world waiting to be explored, and you’re just getting started! Be proud of your progress, and never stop exploring. See you on the next Brighterly adventure!
Frequently Asked Questions on Measuring Angles
Why is a full circle measured as 360 degrees?
The choice of 360 degrees is not arbitrary. It’s believed to originate from ancient astronomers. They noted that the sun moves across the sky by approximately 1 degree each day, and there are roughly 360 days in a year, so they divided the circle into 360 degrees.
What is the difference between a degree and a radian?
Degrees and radians are two different units used to measure angles. A degree is 1/360th of a circle, while a radian is the angle made by taking the radius of a circle and wrapping it along the circle’s edge. Therefore, a full circle is 360 degrees or 2π radians.
Can an angle be negative?
Yes, an angle can be negative. Positive and negative angles are determined based on the direction of rotation. When an angle is measured in the counterclockwise direction, it’s considered positive. When measured in the clockwise direction, it’s considered negative.
What are the differences between complementary and supplementary angles?
Complementary angles are two angles whose measures add up to 90 degrees. They complete each other to form a right angle. On the other hand, supplementary angles are two angles whose measures add up to 180 degrees, forming a straight angle when combined.
How can I measure an angle without a protractor?
While a protractor is a common tool for measuring angles, there are other methods. For example, in a right-angled triangle, you can use trigonometric ratios to calculate angles. Additionally, you can use properties of other angle pairs like complementary, supplementary, vertical, or adjacent angles to calculate unknown angles.
Information Sources
This article was crafted using trusted and well-respected sources to provide you with accurate and engaging information. Here are the primary references we used: