Comparing Fractions – Methods, Definition With Examples
Updated on January 15, 2024
Welcome to our comprehensive guide on Comparing Fractions, a key concept in math for kids. This guide, designed for young learners, aims to simplify and demystify the process of comparing fractions. As a reliable math tutor for kids, we understand the importance of foundational math skills. Through this article, children will learn effective methods to compare fractions, fostering their confidence and interest in online math for kids.
What Are Fractions?
Fractions are a way of representing numbers that are not whole. A fraction consists of two parts: a numerator (top number) and a denominator (bottom number). For example, in the fraction 3/4, 3 is the numerator, showing how many parts we have, and 4 is the denominator, indicating the total number of equal parts in the whole. Understanding fractions is a crucial step in mastering math for kids.
Why Compare Fractions?
Comparing fractions is essential in many real-world situations, such as in cooking or when sharing things fairly among friends. Learning how to compare fractions is not only a vital skill in math but also helps kids develop logical thinking and problem-solving skills, which are integral parts of online math for kids.
Basic Concepts in Fractions
To effectively compare fractions, it’s important to grasp the basic concepts. The numerator represents the number of parts being considered, while the denominator signifies the total number of equal parts. For example, in ½, the numerator 1 tells us we have one part out of a total of 2 parts. These concepts are fundamental in math for kids.
Types of Fractions
Understanding different types of fractions is crucial:
- Proper Fractions: The numerator is smaller than the denominator (e.g., 3/5).
- Improper Fractions: The numerator is greater than the denominator (e.g., 7/4).
- Mixed Numbers: A combination of a whole number and a fraction (e.g., 2 ⅓).
Methods for Comparing Fractions
There are several methods for comparing fractions, each useful in different scenarios.
Same Denominator Method
When fractions have the same denominator, we compare the numerators directly. For instance, in comparing 3/7 and 4/7, since the denominators are the same, we look at the numerators. Here, 4/7 is greater because 4 is more than 3.
Same Numerator Method
If fractions have the same numerator, the fraction with the smaller denominator is larger. For example, compare 4/5 and 4/9. Since 4 is the numerator for both, 4/5 is larger because 5 is smaller than 9.
Cross-Multiplication Method
This method is used when fractions have different numerators and denominators. Multiply across the fractions and compare the results. For instance, to compare 2/3 and 3/4, cross-multiply and compare the products.
Comparing Fractions with Unlike Denominators
Comparing fractions with different denominators can be challenging but is a key skill in math for kids.
Finding Common Denominators
Find a common denominator, then convert each fraction to an equivalent fraction with this common denominator. For example, to compare 1/4 and 2/3, find a common denominator, like 12, and convert both fractions accordingly.
Decimal Conversion Method
Converting fractions to decimals is another method. Divide the numerator by the denominator and compare the decimal values. This method is often used in math for kids programs to simplify comparisons.
Advanced Methods in Comparing Fractions
For a deeper understanding, we explore advanced methods:
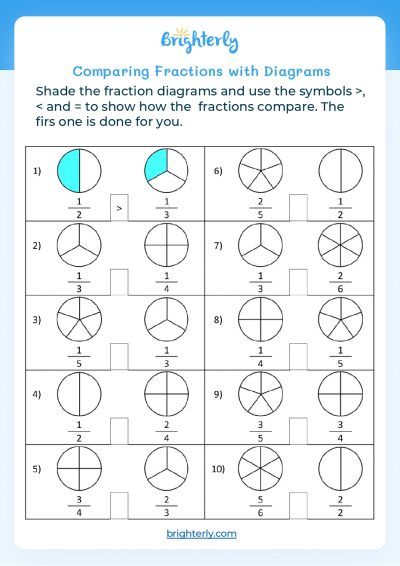
Visualization Techniques
Visual aids like pie charts or number lines can be very helpful, especially in online math for kids. They allow children to see the fractions and understand their sizes relative to each other.
Using Proportions
Setting two fractions equal to each other and solving for an unknown variable is another advanced method. This is particularly useful in real-world applications.
Practical Examples and Exercises
In this section, we’ll go through some practical examples and exercises to help reinforce the concepts of comparing fractions. These examples are designed to be engaging and relatable for kids, making the learning process more effective and enjoyable.
Example 1: Baking Cookies
Imagine you are baking cookies and the recipe requires 3/4 cup of sugar. However, you only have a 1/4 cup measuring cup. How many times will you need to fill the 1/4 cup to have enough sugar for the recipe? The answer is 3 times because 3/4 divided by 1/4 equals 3.
Exercise 1
Which is larger: 3/8 or 1/2? To compare, find a common denominator or convert them to decimals. Here, 1/2 can be seen as 4/8, so now it’s clear that 1/2 (or 4/8) is larger than 3/8.
Example 2: Sharing Pizza
You and your two friends are sharing a pizza cut into 8 equal slices. You eat 3/8 of the pizza, and one friend eats 2/8. Who ate more pizza? Since 3/8 is greater than 2/8, you ate more pizza.
Exercise 2
Compare 5/6 and 2/3 using the cross-multiplication method. Multiply 5 by 3 and 6 by 2, getting 15 and 12, respectively. Since 15 is greater than 12, 5/6 is larger than 2/3.
Example 3: Lemonade Stand
If you have a lemonade stand and sell 2/5 of your lemonade in the morning and 1/5 in the afternoon, what fraction of your lemonade did you sell in total? By adding 2/5 and 1/5, you find that you sold 3/5 of your lemonade.
Exercise 3
Determine which is smaller: 7/10 or 3/4. Convert both to decimals or find a common denominator to compare. Converting to decimals, 7/10 is 0.7 and 3/4 is 0.75. Therefore, 7/10 is smaller than 3/4.
These examples and exercises are a great way to practice comparing fractions. They illustrate how fractions are used in everyday situations, making the concept more relatable and easier to grasp for kids. Remember, the key to mastering fractions is practice and application in real-life scenarios.
FAQs on Comparing Fractions
What is the easiest method to compare fractions?
The easiest method varies depending on the fractions. For similar denominators, use the same denominator method; for similar numerators, use the same numerator method.
Can fractions be compared visually?
Yes, using visual aids like pie charts or number lines is a great way to compare fractions, especially in online math for kids.
Is finding a common denominator always necessary?
While finding a common denominator is one method, it’s not always necessary. Other methods like decimal conversion can be effective too.
How does Brighterly simplify comparing fractions for kids?
Brighterly, as an online math tutor for kids, uses interactive methods and practical examples to make learning fractions easy and fun.
Online Winter Math Camp for Grades 1-8
Join us to help your kid achieve their full math potential.
Choose kid's grade
Online Winter Math Camp for Grades 1-8
Join our Math Winter Camp and boost your child's math skills with our best tutors.
