Congruent – Definition with Examples
Updated on January 14, 2024
Welcome to Brighterly, where we make math fun and easy for kids! Today, we will learn about the congruence of triangles. Congruent triangles are an essential concept in geometry, and understanding them will help you tackle many mathematical problems. Let’s get started!
Congruent Meaning in Maths
In mathematics, congruent objects have the same size and shape. The term “congruent” comes from the Latin word “congruere,” which means “to come together.” In mathematical terms, it means that two objects fit perfectly into each other, just like two puzzle pieces. For example, if we have two congruent triangles, we can say that all their sides and angles are equal, and they have the same size and shape.
Congruence has various applications in different fields of mathematics, such as geometry, algebra, and trigonometry. In geometry, congruence is used to define congruent figures, which are shapes that are identical in size and shape. In algebra, congruence is used to define congruent numbers, which are integers that have the same remainder when divided by a given modulus. In trigonometry, congruence is used to define congruent triangles, which have the same size and shape.
Congruent Meaning in Geometry
In geometry, congruent figures are shapes that can be transformed into one another through rotations, reflections, or translations. These transformations don’t change the size or shape of the figures, which is why they remain congruent even after being moved or flipped around. In other words, congruent figures have the same size and shape, but they may be in different positions or orientations.
The concept of congruence is essential in geometry, as it helps to define many geometric shapes and their properties. For example, congruent line segments are segments that have the same length, and congruent angles are angles that have the same measure. Congruent circles are circles that have the same radius, and congruent triangles are triangles that have the same size and shape.
Symbol of Congruence
The symbol for congruence is “≅,” which is a combination of two parallel lines. For example, if we have two congruent triangles, we can write it as △ABC ≅ △DEF. This notation tells us that triangle ABC is congruent to triangle DEF.
Congruent Line Segments, Angles, Circles, and Triangles
Congruent line segments are segments that have the same length. We can denote this by writing AB ≅ CD, meaning that line segment AB is congruent to line segment CD. Congruent angles are angles that have the same measure in degrees. We can write this as ∠A ≅ ∠B, meaning that angle A is congruent to angle B. Congruent circles are circles that have the same radius. We can write this as ⊙A ≅ ⊙B, meaning that circle A is congruent to circle B.
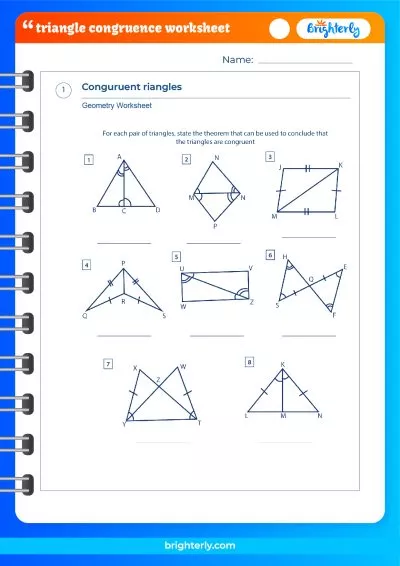
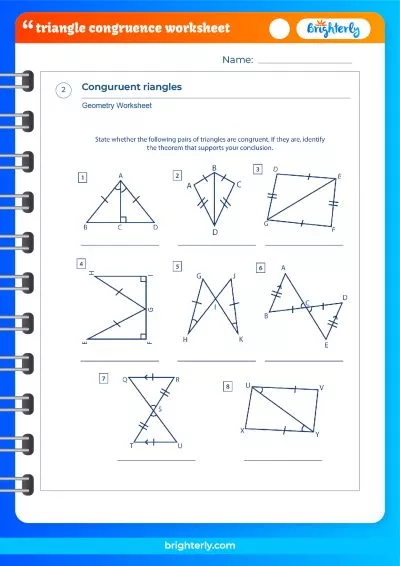
Congruent triangles are triangles that have the same size and shape. Two triangles are congruent if all their corresponding sides and angles are congruent. There are four main postulates, or rules, to determine if two triangles are congruent: SSS, SAS, ASA, and AAS.
In SSS (Side-Side-Side), if all three sides of one triangle are congruent to the corresponding sides of another triangle, then the triangles are congruent. In SAS (Side-Angle-Side), if two sides and the included angle of one triangle are congruent to the corresponding sides and included angle of another triangle, then the triangles are congruent. In ASA (Angle-Side-Angle), if two angles and the included side of one triangle are congruent to the corresponding angles and included side of another triangle, then the triangles are congruent. In AAS (Angle-Angle-Side), if two angles and a non-included side of one triangle are congruent to the corresponding angles and non-included side of another triangle, then the triangles are congruent.
Difference between Congruent Figures and Similar Figures
While congruent figures have the same size and shape, similar figures have the same shape but not necessarily the same size. Think of similar figures as scaled versions of each other, like a smaller or larger copy of the same picture. In other words, two figures are similar if they have the same shape but are not necessarily the same size.
CPCT Rules in Maths
CPCT stands for Corresponding Parts of Congruent Triangles. It means that if two triangles are congruent, their corresponding parts (sides and angles) are also congruent. This property is useful in solving problems that involve congruent triangles.
Solved Examples
Let’s go through some solved examples to better understand the concept of congruent triangles.
Example 1: In triangle ABC, AB = 4 cm, BC = 6 cm, and AC = 8 cm. In triangle DEF, DE = 4 cm, EF = 6 cm, and DF = 8 cm. Are the two triangles congruent?
Solution: We can use the SSS postulate to check if the triangles are congruent. Since AB = DE, BC = EF, and AC = DF, we can conclude that the two triangles are congruent.
Example 2: In triangle PQR, PQ = 5 cm, QR = 7 cm, and RP = 6 cm. In triangle XYZ, XY = 8 cm, YZ = 10 cm, and ZX = 6 cm. Are the two triangles congruent?
Solution: We can use the SAS postulate to check if the triangles are congruent. Since PQ = XY, RP = XZ, and ∠PQR = ∠XYZ, we can conclude that the two triangles are congruent.
Practice Problems
Now that we’ve gone through some examples, it’s time to practice on your own! Try these problems to test your understanding of congruent triangles.
-
In triangle ABC, AB = 6 cm, BC = 8 cm, and AC = 10 cm. In triangle DEF, DE = 5 cm, EF = 7 cm, and DF = 9 cm. Are the two triangles congruent? If yes, which postulate did you use?
-
In triangle LMN, LM = 12 cm, MN = 10 cm, and NL = 8 cm. In triangle XYZ, XY = 16 cm, YZ = 13 cm, and ZX = 10 cm. Are the two triangles congruent? If yes, which postulate did you use?
-
In triangle PQR, PQ = 9 cm, QR = 12 cm, and RP = 15 cm. In triangle STU, ST = 6 cm, TU = 8 cm, and US = 10 cm. Are the two triangles congruent? If yes, which postulate did you use?
Conclusion
Congruent triangles are an important concept in geometry, and understanding them can help you solve many mathematical problems. Remember, two triangles are congruent if all their corresponding sides and angles are congruent. There are four main postulates to determine if two triangles are congruent: SSS, SAS, ASA, and AAS. Keep practicing, and you’ll be a pro at congruent triangles in no time!
Frequently Asked Questions on Congruent
What is the meaning of CPCT in maths?
CPCT stands for Corresponding Parts of Congruent Triangles. When two triangles are congruent, their corresponding parts (sides, angles, vertices) are also congruent. This is a fundamental concept in geometry that allows us to compare and analyze different parts of congruent triangles. The CPCT rule is often used in solving problems involving congruent triangles, as it provides a shortcut to identify corresponding parts that are congruent.
What are the four postulates to determine if two triangles are congruent?
The four postulates, or rules, to determine if two triangles are congruent are:
-
SSS (Side-Side-Side): If all three sides of one triangle are congruent to the corresponding sides of another triangle, then the triangles are congruent.
-
SAS (Side-Angle-Side): If two sides and the included angle of one triangle are congruent to the corresponding sides and included angle of another triangle, then the triangles are congruent.
-
ASA (Angle-Side-Angle): If two angles and the included side of one triangle are congruent to the corresponding angles and included side of another triangle, then the triangles are congruent.
-
AAS (Angle-Angle-Side): If two angles and a non-included side of one triangle are congruent to the corresponding angles and non-included side of another triangle, then the triangles are congruent.
These postulates are used to prove that two triangles are congruent, and they are based on the fact that corresponding sides and angles of congruent triangles are equal.
What is the difference between congruent and similar figures?
Congruent figures are shapes that have the same size and shape. Two figures are congruent if all their corresponding sides and angles are equal. Congruent figures can be transformed into each other through rotations, reflections, or translations, without changing their size or shape. Similar figures, on the other hand, have the same shape but may be different in size. Two figures are similar if they have the same shape, but their corresponding sides are proportional in length. In other words, one figure is a scaled version of the other. The concept of similarity is often used in geometry to compare the shapes of different objects.
What is the symbol for congruence?
The symbol for congruence is “≅”, which is a combination of two parallel lines. This symbol is used to denote that two geometric figures are congruent, meaning they have the same size and shape. For example, if we have two congruent triangles, we can write it as △ABC ≅ △DEF. This tells us that triangle ABC is congruent to triangle DEF.
Why is congruence important in geometry?
Congruence is important in geometry because it helps to define many geometric shapes and their properties. Congruent figures have the same size and shape, which allows for accurate measurement and analysis of geometric properties. By identifying corresponding parts of congruent triangles, we can compare and analyze different parts of geometric figures. Congruence is also used in geometry to solve problems involving angles, sides, and other geometric properties of shapes. In addition, the concept of congruence is fundamental to many geometric theorems and proofs.
Troubles with Geometry?
- Does your child struggle with understanding the basics of geometry?
- Try lessons with an online tutor.
Choose kid's grade
Is your child having difficulties with mastering geometry fundamentals? An online tutor could provide the necessary support.
