Dimes – Definition With Examples
reviewed by Jo-ann Caballes
Updated on October 4, 2024
Knowing the values of currencies is necessary to manage one’s finances effectively. This article covers the difference between a nickel and a dime, how nickels and dimes compare to dollars, and real-world coin counting and conversion.
The Current System of Money
The first monetary units were based on the weight of rocks. Human understanding and abilities were polished as time went on. The first metal money was introduced in about 1000 BCE. The coin was made from bronze and copper.
Like in the past, modern coins are made from gold and silver, adding to the paper money today. Different nations use various systems. For instance, Indians use the rupee, the Chinese use the yen, and the British use the Pound as their official currency. Also, almost all European countries use the Euro.
What Is Dime?
The word Dime came from the Latin word “decimus” which represents anything split into ten (or a tenth part). Later, in France, the word for representing tenths changed to “disme.” Fast-forward to the present day, and we refer to money using decimal arithmetic after the first dime was minted in 1792. Today, the American dime is equal to ten cents, and the ratio of 1 dime to dollar is 1:10. It helps people carry fewer coins in their purses when giving out change.
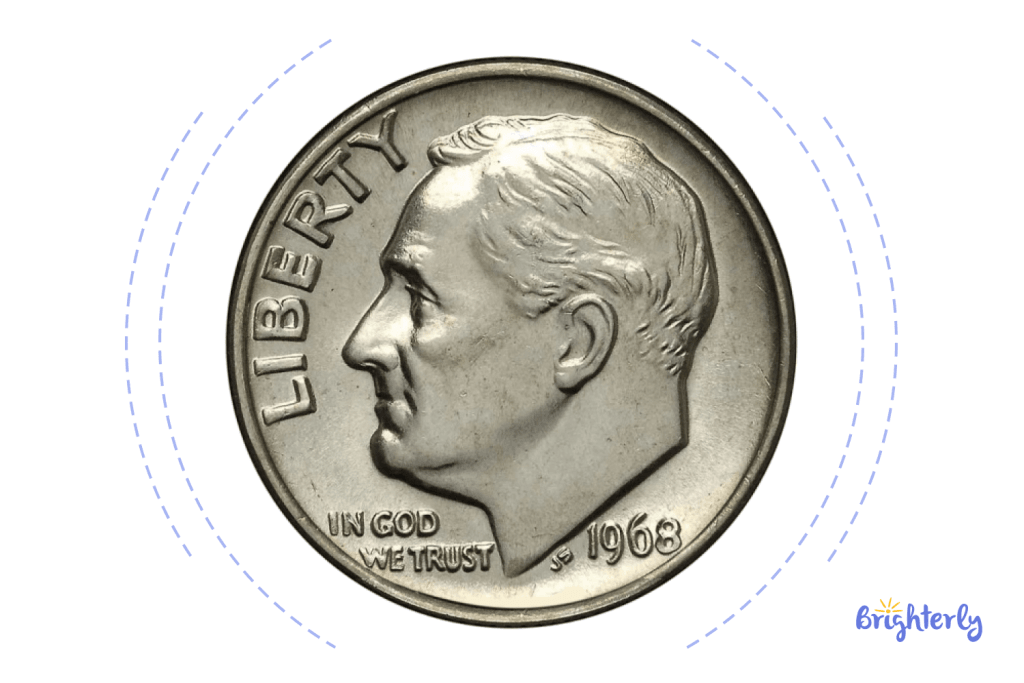
Penny and Dime
While many other coins are used and valued in the US, two of the most prevalent and extensively used are the dime and the penny. Unlike the nickel vs dime comparison, the penny is the most basic unit of money in the US. It has the same value as the cent and can be used interchangeably. Its front picture of Abraham Lincoln and copper color make it stand out.
Seeing as the dime is ten cents, it is also worth ten pennies. Although the penny may not be worth much, it is often the first currency that children encounter when they begin to learn about money. As a parent or tutor, encourage kids to save even the smallest sums over time and teach them to count.
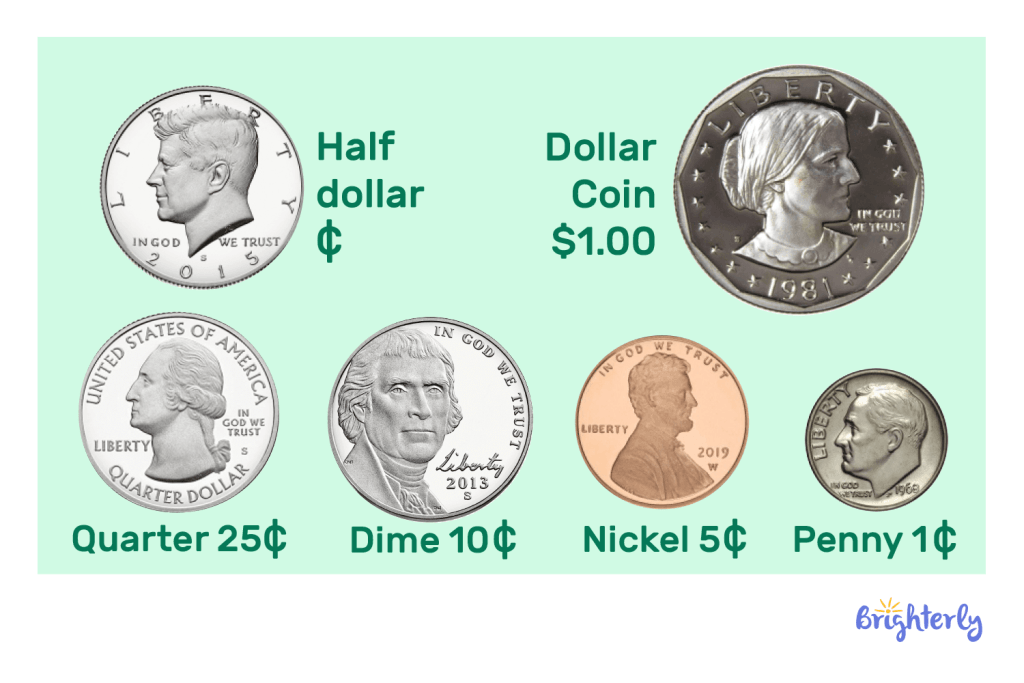
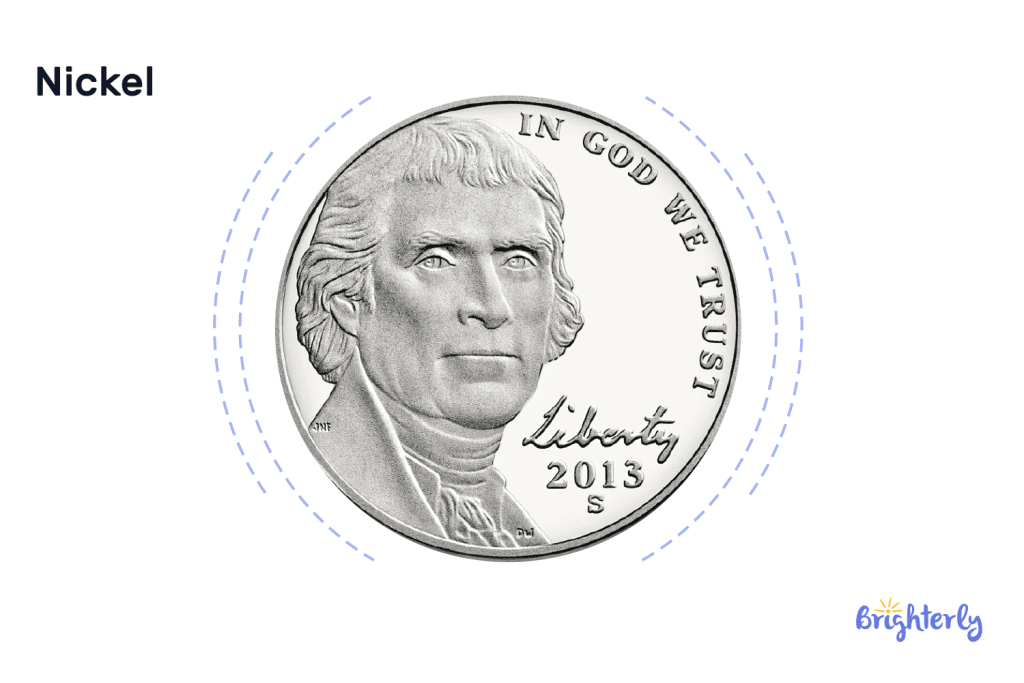
How Much is a Dime and a Nickel?
This question will come up in classrooms when teaching first and second-graders about money. The nickel is worth five cents or half of a dime, making it less valuable. You may get precise change or round-up transactions using nickel coins, while dimes are used for minor purchases or sales tax.
Also, your students may ask you, “Are nickels bigger than dimes?” Tell them that a nickel is larger and thicker than a dime, even though a dime is worth more. Bring out both coins and encourage them to look closely; they’ll see that a dime is smaller and lighter than a nickel.
How Much Dimes Make a Dollar?
If you are wondering, “Does 10 dimes make a dollar?” you’re right, it does. The dollar is worth one hundred cents or ten dimes. Knowing the difference between two coins may help you manage your money better.
The dollar and the dime are two distinct but complementary types of money. Dimes may be used for little purchases or steady savings but the dollar is much more valuable. While most people prefer to use dollars for bigger purchases, you can also use dimes.
How Many Cents is One Dime?
When considering the number of cents in a dime, note that the latter is a 10-cent coin. Even though dimes are smaller, they’re more valuable than both nickels and pennies.
Counting Dimes and Other Coins
The ability to count money is an essential skill to have and makes computations easier. For instance, if you have five dimes, you may add them up using the tens system: 10, 20, 30, 40, 50. This gives you fifty cents.
But knowing how to count dimes isn’t enough; being able to count pennies, nickels, and quarters is just as crucial. The worth of these coins varies:
- You count pennies by ones because each one is worth one cent. For instance, four pennies are equivalent to four cents.
- You can count nickels by fives since each one is worth five cents. For example, if you have three nickels, it means fifteen cents.
- You count the quarters by twenty-fives because each quarter is worth twenty-five cents. Two quarters, for instance, is equivalent to fifty cents.
When you have a mix of dimes and other coins, you must properly combine their values to get the total amount.
For instance, if you have 2 dimes and 3 pennies, count 10 + 10 = 20 cents. Add the pennies: 20 + 1 + 1 + 1 = 23 cents.
Total: 23 cents.
Coins of varying values, like quarters, dimes, nickels, and pennies, may be easily counted if arranged in ascending value order. This will show the clear difference between a dime and a cent, for instance.
Completing the sum by hand or in your head is a good way to guarantee precision. With consistent practice, you will count more easily, regardless of the currency mix.
Solved Math Tasks: Examples
Question 1
James has 10 nickels. He wants to trade them in for dimes. How many dimes will he receive in exchange for his 10 nickels?
Solution
To convert 10 nickels into dimes:
Value of All Nickels:
- 10 nickels x 5¢ = 50¢.
Value of each dime:
- 10¢.
Number of Dimes:
- 50 ¢ ÷ 10 ¢ = 5 dimes.
| So, James will have 5 dimes. |
Question 2
A bag contains n nickels worth the same amount as 3 dimes. Find the value of n.
Outcome
Value of Three Dimes:
- 3 x 10 ¢ = 30 ¢.
Amount of Each Nickel:
- Five cents.
Number of Nickels:
- 30 ¢ ÷ 5 ¢ = 6 nickels.
| So, 6 is the value of n. |
Practice Math Problems
Money Worksheets
Now that your students know what a dime is and can answer questions like is 10 dimes a dollar, take them to the next stage of learning about money. Use Brighterly’s worksheets to make them practice counting and using dimes and become money experts.
- Counting dimes worksheets
- Counting quarters worksheets
- Counting pennies worksheets
- Counting money worksheets
Troubles with Geometry?
- Does your child need additional support with mastering the concept of geometry?
- Start lessons with an online tutor.
Choose kid's grade
Is your child finding it challenging to understand geometry concepts? An online tutor could be the answer.
