Dividing Fractions – Definition with Examples
Updated on January 7, 2024
Division of fractions might appear intimidating to some young minds, but with the right guidance and resources, it can be easily grasped and mastered. At Brighterly, we are dedicated to helping children overcome their fear of math and to unlock their full potential. Our unique approach to teaching mathematics makes the subject both enjoyable and approachable, enabling children to embrace the beauty of dividing fractions and to confidently tackle more complex mathematical problems in the future.
To excel in the division of fractions, it is crucial to understand the underlying principles and the step-by-step process involved. With patience, practice, and the right guidance from our dedicated team of experts at Brighterly, children can excel in this fundamental area of mathematics and lay a strong foundation for their future endeavors.
What is Meant by Dividing Fractions?
Dividing fractions is a fundamental operation in mathematics where one fraction is divided by another. This concept is vital for young learners, as it lays the groundwork for tackling more advanced mathematical problems later on. In essence, dividing fractions is determining how many times one fraction can fit into another. At Brighterly, we strive to present this seemingly complex concept in an engaging and simplified manner, allowing children to develop a strong foundation in this area of mathematics.
How to Divide Fractions?
To divide fractions, one must employ a straightforward technique – multiplying by the reciprocal. The reciprocal of a fraction is obtained by inverting it, swapping the numerator and denominator. For instance, the reciprocal of 3/4 is 4/3. This method is an essential part of the Brighterly approach, making the division of fractions an easy-to-understand process for young learners.
If you are looking to improve your understanding of dividing fractions, then the math worksheets Brighterly are an excellent resource to consider. These worksheets are specifically designed to help you learn and reinforce your knowledge of this concept.
Steps to Divide Fractions
- Identify the problem: Note down the two fractions involved in the division problem.
- Determine the reciprocal: Find the reciprocal of the divisor (the second fraction).
- Perform multiplication: Multiply the dividend (the first fraction) by the reciprocal of the divisor.
- Simplify: If possible, simplify the resulting fraction for easier understanding.
Properties of Dividing Fractions
- Dividing by a fraction is equivalent to multiplying by its reciprocal, which simplifies the process.
- The division of fractions is commutative, meaning that the order in which the fractions are divided does not influence the result.
- The division of fractions is associative, implying that the grouping of the fractions does not affect the outcome.
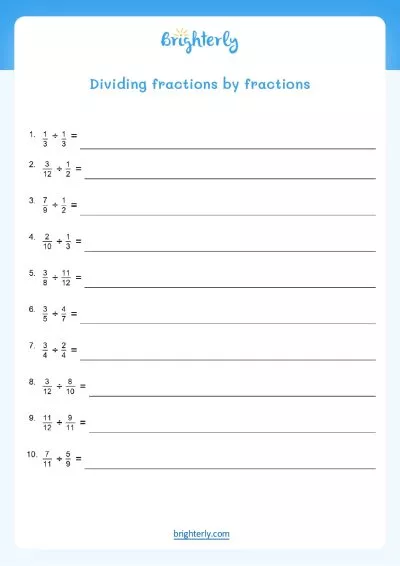
Dividing Fractions by Fractions
To divide fractions by fractions, follow the steps outlined above. For example, let’s divide 1/2 by 3/4:
- Identify the problem: 1/2 ÷ 3/4
- Determine the reciprocal: The reciprocal of 3/4 is 4/3.
- Perform multiplication: 1/2 × 4/3 = 4/6
- Simplify: 4/6 simplifies to 2/3.
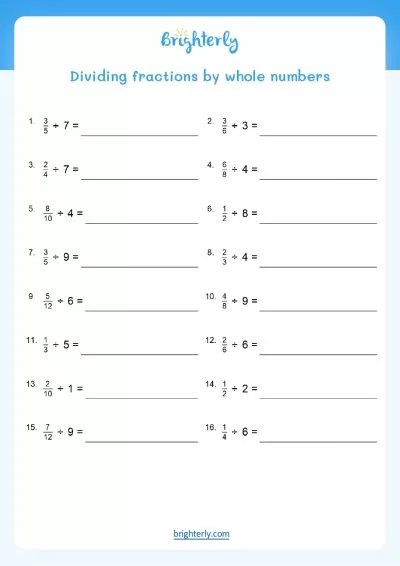
Division of Fractions with Whole Numbers
When dividing a fraction by a whole number, convert the whole number to a fraction before proceeding with the steps mentioned above. For instance, if you are dividing 2/3 by 4, convert 4 to 4/1 and continue with the division.
Dividing Fractions with Decimals
To divide fractions with decimals, first convert the decimal to a fraction, and then follow the steps for dividing fractions. For example, to divide 3/5 by 0.2, convert 0.2 to 1/5 and proceed with the division.
Division of Fractions and Mixed Numbers
When dividing fractions and mixed numbers, first convert the mixed numbers into improper fractions before following the steps for dividing fractions. For instance, if you are dividing 2 1/2 by 1 1/4, convert the mixed numbers to improper fractions (5/2 and 5/4) and proceed with the division.
By incorporating these techniques into the Brighterly approach, we help children develop a strong foundation in dividing fractions, paving the way for their future mathematical success.
Solved Examples on Dividing Fractions
To better understand the division of fractions, let’s explore some solved examples, using the Brighterly approach to make the process engaging and clear for young learners.
Example 1: Divide 3/4 by 2/3:
3/4 ÷ 2/3 = 3/4 × 3/2 = 9/8
Example 2: Divide 5/6 by 4:
5/6 ÷ 4 = 5/6 × 1/4 = 5/24
Example 3: Divide 7/9 by 1/3:
7/9 ÷ 1/3 = 7/9 × 3/1 = 21/9 = 7/3
Practice Questions on Dividing Fractions
Challenge yourself with these practice questions, designed to help reinforce the concepts and techniques we’ve covered. Remember to apply the Brighterly approach to make the process more enjoyable and engaging.
- Divide 7/8 by 1/2
- Divide 3/5 by 0.4
- Divide 2 3/4 by 1 1/2
- Divide 4/9 by 5/6
- Divide 5/7 by 2/3
By working through these examples and practice questions, young learners can develop a strong understanding of dividing fractions, setting them up for success in more advanced mathematical concepts. At Brighterly, we believe that every child has the potential to excel in mathematics, and our mission is to help them achieve this potential by making the learning process engaging and enjoyable.
Conclusion
Developing a strong grasp of the division of fractions is an indispensable skill for children as they embark on their mathematical journey. By embracing the Brighterly approach, young learners can follow the straightforward steps and gain a deep understanding of the properties of dividing fractions, empowering them to tackle these problems effortlessly and with confidence.
At Brighterly, we believe that every child has the potential to excel in mathematics, and our mission is to nurture this potential by making math accessible, engaging, and enjoyable. By mastering the division of fractions, children can build a strong foundation in mathematics, preparing them for more advanced topics and empowering them to approach future challenges with enthusiasm and determination.
Frequently Asked Questions on Dividing Fractions
Why do we multiply by the reciprocal when dividing fractions?
Multiplying by the reciprocal is a helpful trick that simplifies the division process. This method is based on the principle that dividing by a number is the same as multiplying by its reciprocal. By using this technique, we can transform the division of fractions into a multiplication problem, making it easier for young learners to understand and solve. At Brighterly, we incorporate this approach to make the concept of dividing fractions more accessible and engaging for children.
Can you divide fractions with different denominators?
Yes, you can divide fractions with different denominators by following the steps mentioned above. When dividing fractions, there is no need to find a common denominator, as is the case when adding or subtracting fractions. This is because the process of dividing fractions involves multiplying by the reciprocal, which does not require the fractions to have the same denominator. At Brighterly, we emphasize this distinction to help children differentiate between various fraction operations and develop a solid understanding of each.
How do you divide fractions with mixed numbers?
To divide fractions with mixed numbers, first, convert the mixed numbers into improper fractions. Improper fractions have a larger numerator than the denominator, making them easier to work with when dividing fractions. Once you’ve converted the mixed numbers to improper fractions, follow the steps for dividing fractions as described above. By breaking down the process into manageable steps, Brighterly helps young learners build confidence and master the skill of dividing fractions with mixed numbers.
Information Sources
Troubles with Fractions?
- Does your child struggle to master fractions basics?
- Try learning with an online tutor.
Choose kid's grade
Is your child having trouble grasping the basics of fractions? An online tutor could provide the necessary assistance.
