• Math for kids
• >
• Knowledge Base
• >
• Division – Definition, Properties, FAQs, Examples

Division – Definition, Properties, FAQs, Examples

Division, a foundational operation in mathematics, significantly impacts various aspects of our day-to-day lives. In this comprehensive guide by Brighterly, we invite young learners to embark on an exciting journey to explore the world of division. We will delve into the principles behind division, examine its rules and symbols, and unravel the fascinating properties that govern this essential mathematical operation.

With Brighterly’s interactive approach, we aim to stimulate young minds by providing them with engaging practice problems and real-life examples that illustrate the practical applications of division. By making the learning process enjoyable and relatable, we strive to foster a lasting interest in mathematics and inspire children to unlock their full potential.

What is Division?

Division is a crucial mathematical operation that allows us to figure out how many times one quantity fits inside another. It serves as the counterpoint to multiplication, making it possible to split larger quantities into smaller, equal portions. Division is a versatile tool that can be applied to various aspects of our lives, from distributing items evenly among a group to determining the mean of a dataset. Whether we’re sharing a pizza with friends or calculating an athlete’s average performance, division is an indispensable operation for making sense of the world around us.

Mathematical Notation for Division

In the realm of mathematics, division can be denoted in several ways. The two most prevalent notations are the forward slash (/) and the division sign (÷). For instance, if we aim to divide the number 8 by 2, we can express this operation as either 8 ÷ 2 or 8/2. While these notations may appear different, they are both equally valid and convey the same intent.

Division can also be indicated using a horizontal line with the dividend placed above and the divisor below, known as a fraction bar. This representation is particularly useful when dealing with fractions or more complex expressions. In our previous example, the division of 8 by 2 could be written as a fraction: 8/2. Despite the differences in appearance, all of these notations are employed to communicate the same mathematical concept: division.

Division Rule

The fundamental rule of division stipulates that when you divide a number (the dividend) by another number (the divisor), the outcome is called the quotient. In some instances, there may be a remainder—a portion of the dividend that cannot be evenly divided by the divisor. It is important to remember that the divisor should never be zero, as division by zero is an undefined operation in mathematics, leading to paradoxical results and logical inconsistencies.

Division Symbol

The division symbol, ÷, serves as a visual representation of the division operation within a mathematical equation. Also known as the obelus, this symbol was first introduced as a division symbol by Swiss mathematician Johann Rahn in 1659. Since then, the division symbol has become a universally recognized method for indicating the process of dividing one number by another, enabling clear communication and understanding among mathematicians and students alike.

Division Algorithm

The division algorithm encompasses a range of step-by-step procedures for finding the quotient and remainder when dividing two numbers. Several methods can be employed to perform division, such as long division, short division, and synthetic division. Each technique has its own set of advantages and is suited to different levels of complexity and learner understanding.

• Long division is a standard method for dividing larger numbers or decimals. This technique requires the systematic application of basic arithmetic operations—multiplication, subtraction, and sometimes addition—until the entire dividend has been divided by the divisor.
• Short division, also known as “bus stop” or “chunking” method, is a more informal approach, particularly suited for simpler problems or when the divisor is a single-digit number. This method relies on mental math and estimation to arrive at the quotient and remainder.
• Synthetic division is a specialized technique used primarily for dividing polynomials, streamlining the long division process for this specific type of problem.

The selection of the most appropriate division algorithm depends on the nature of the problem and the learner’s level of mathematical understanding. Each method offers a unique approach to tackling division, catering to the diverse needs and abilities of kids.

Special Names for Numbers in Division Equation

In a division equation, there are three main components:

1. Dividend: The number being divided.
2. Divisor: The number by which the dividend is being divided.
3. Quotient: The result obtained after division.
4. Remainder: The part of the dividend that is not divisible by the divisor.

For example, in the equation 15 ÷ 3 = 5, 15 is the dividend, 3 is the divisor, 5 is the quotient, and there is no remainder.

Properties of Division

Division has several interesting properties, including:

1. Division by 1: When a number is divided by 1, the quotient is always equal to the dividend.
2. Division by 0: Division by zero is undefined in mathematics.
3. Commutative property: Division does not follow the commutative property, which means a ÷ b ≠ b ÷ a.
4. Associative property: Division is not associative, meaning (a ÷ b) ÷ c ≠ a ÷ (b ÷ c).

Practice Problems On Division

Sharpen your division skills with these practice problems:

1. 24 ÷ 6 = ?
2. 35 ÷ 5 = ?
3. 42 ÷ 7 = ?
4. 18 ÷ 3 = ?
5. 27 ÷ 9 = ?

Looking for math worksheets for kids? Check out Brighterly Math for Kids! We have a wide variety of tasks on any topic that will help your child learn and excel in math.

Division Examples

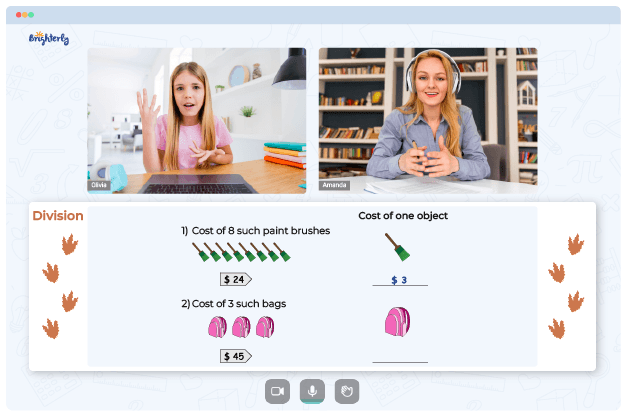
Division is everywhere in our daily lives! Here are a few examples:

1. Sharing a pizza among friends: If you have a pizza with 8 slices and want to share it equally among 4 friends, you would divide the total number of slices (8) by the number of friends (4) to find out how many slices each person gets. So, 8 ÷ 4 = 2. Each friend gets 2 slices of pizza.

   2. Calculating the average: If a student scores 80, 90, and 100 on three tests, you can find the average by dividing the sum of the scores by the number of tests. The sum is 80 + 90 + 100 = 270, and there are 3 tests. Therefore, the average score is 270 ÷ 3 = 90.

   3. Distributing chores: In a family of 5, if there are 10 chores to be done over the weekend, you can divide the total chores by the number of family members to find out how many chores each person should do. So, 10 ÷ 5 = 2. Each family member should complete 2 chores.

Conclusion

Division, a fundamental mathematical operation, empowers us to dismantle sizable quantities into more manageable portions, distribute resources fairly, and determine averages. By embracing the concepts of division and its properties, students at Brighterly can not only elevate their math skills but also unlock their potential to tackle real-world challenges with confidence and dexterity.

Brighterly’s engaging approach to teaching division provides a solid foundation for students to develop their problem-solving abilities. As students progress through various division techniques and strategies, they will gain a deeper appreciation for the power and versatility of this essential operation. By understanding how division works and applying it to everyday scenarios, they can approach complex problems with a greater sense of creativity and resourcefulness.

In conclusion, mastering division is not only a valuable skill for excelling in math but also an indispensable tool for navigating the complexities of life. By learning division through Brighterly’s comprehensive and engaging curriculum, students are equipped with the knowledge and confidence to tackle an array of challenges, both within the realm of mathematics and beyond.

Frequently Asked Questions on Division

What is division?

Division is a mathematical operation that helps determine how many times one number can be contained within another number.

What are the special names for numbers in a division equation?

The special names are dividend (the number being divided), divisor (the number by which the dividend is being divided), quotient (the result obtained after division), and remainder (the part of the dividend that is not divisible by the divisor).

Can you divide by zero?

No, division by zero is undefined in mathematics.

What are the properties of division?

Division has several properties, such as division by 1, division by 0 (undefined), non-commutative, and non-associative.

Information Sources

To learn more about division, you can refer to the following resources:

1. Division – Wikipedia
2. Division – Math is Fun
3. Division – Khan Academy