Isosceles Triangles – Definition, Parts, Types, Properties
Updated on January 6, 2024
Welcome to Brighterly – the ultimate destination for kids who love to explore the magical world of mathematics! At Brighterly, our mission is to make learning math a joyful and exciting experience for children of all ages. Today, we’re going to embark on an exciting journey into the fascinating world of Isosceles Triangles. With our unique, interactive, and colorful approach, we’ll make this topic easy to understand and enjoyable to learn. So, put on your thinking caps, and let’s dive right into the realm of isosceles triangles together!
At Brighterly, we believe that math should be accessible, engaging, and enjoyable for everyone, and we’re committed to making that a reality for children everywhere. So, join us on this fantastic voyage into the captivating universe of isosceles triangles, and together we’ll uncover their secrets, learn about their properties, and discover how they fit into the grand tapestry of mathematics. And remember, with Brighterly, the sky’s the limit when it comes to unlocking your full potential in the world of math!
What is an Isosceles Triangle?
An isosceles triangle is a remarkable and versatile type of triangle that boasts two sides of equal length. This extraordinary characteristic sets it apart from its cousins – the scalene and equilateral triangles. You might be surprised to learn that isosceles triangles can be found all around us, from awe-inspiring architectural marvels to captivating works of art, and even in the intricate patterns of nature. With such a widespread presence, it’s crucial for kids to learn about isosceles triangles and grasp their unique properties to develop a strong foundation in geometry.
Isosceles Triangle Definition
In the realm of geometry, an isosceles triangle is defined as a triangle that has at least two sides of equal length. These congruent sides are referred to as the legs, while the third, distinct side is called the base. The point where the equal sides converge is known as the vertex, creating a distinctive V-shape. The angles that are formed between the equal sides and the base are called the base angles, and they share an essential characteristic: they are always equal in measure. This fascinating relationship between the sides and angles of an isosceles triangle makes it a captivating topic for young learners to explore and understand.
Properties of an Isosceles Triangle: A Closer Look
An isosceles triangle possesses several fascinating properties that make it a unique and captivating figure in geometry. Let’s delve deeper into these properties to gain a better understanding of this remarkable shape:
-
Equal sides: At the heart of an isosceles triangle lies its most defining characteristic: at least two sides boast equal length. This symmetry imbues the triangle with a sense of balance and harmony.
-
Equal angles: In perfect harmony with the equal sides, the base angles (the angles opposite the equal sides) are always congruent. This congruence is not just a coincidence but a fundamental property of isosceles triangles that holds true regardless of their size or proportions.
-
Symmetry: An isosceles triangle has an inherent line of symmetry along its vertical axis, which passes through the vertex. This symmetry gives the triangle a visually pleasing appearance and provides the basis for many fascinating mathematical explorations.
Angles of Isosceles Triangle: A Comprehensive Breakdown
In an isosceles triangle, the two base angles are always equal in measure. The vertex angle (the angle between the two equal sides) can exhibit a wide range of values. However, one crucial fact remains constant: the sum of all three angles in any triangle will always equal 180 degrees. Armed with this knowledge, you can effortlessly calculate the vertex angle by subtracting the sum of the base angles from 180 degrees.
Isosceles Triangle Theorem: A Cornerstone of Geometry
The Isosceles Triangle Theorem is a fundamental principle in geometry that states if two sides of a triangle are congruent, then the angles opposite those sides are also congruent. This theorem is not only essential for understanding isosceles triangles but also plays a crucial role in identifying and classifying triangles based on their sides and angles.
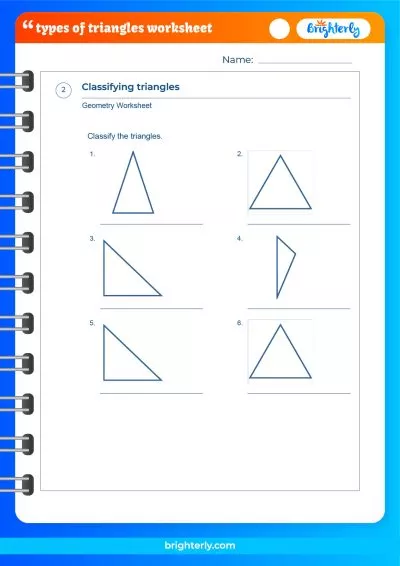
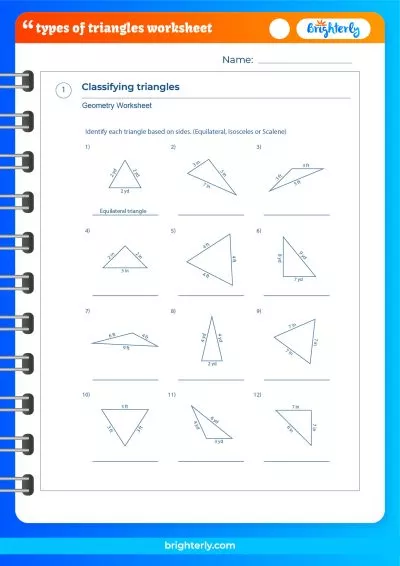
Scalene, Equilateral, and Isosceles Triangle: The Three Pillars of Triangular Geometry
Triangles can be classified into three main categories based on their sides, each with its unique characteristics:
-
Scalene Triangle: A triangle where all sides have different lengths, resulting in three distinct angles.
-
Equilateral Triangle: A triangle with all sides of equal length, creating three congruent angles of 60 degrees each.
-
Isosceles Triangle: A triangle with at least two sides of equal length, giving rise to two congruent base angles and a distinct vertex angle.
These three types of triangles form the foundation of triangular geometry, offering endless opportunities for exploration, discovery, and mathematical creativity.
Exploring the Types of Isosceles Triangles
Isosceles triangles exhibit a rich diversity, further branching into two subtypes based on their vertex angle. Understanding these subtypes allows us to appreciate the vast range of shapes and forms that isosceles triangles can take:
-
Acute Isosceles Triangle: In this type of isosceles triangle, the vertex angle measures less than 90 degrees. The acute vertex angle gives the triangle a sharp, slender appearance, making it a popular choice for various geometric designs and patterns.
-
Obtuse Isosceles Triangle: An obtuse isosceles triangle features a vertex angle greater than 90 degrees. The larger vertex angle creates a more spread-out, expansive shape that offers a stark contrast to the acute isosceles triangle.
Unraveling the Secrets of Isosceles Triangle: Area and Perimeter
To fully appreciate the beauty and versatility of isosceles triangles, it’s essential to understand how to calculate their area and perimeter. These calculations are key to solving various geometric problems and unlocking the hidden potential of these unique triangles.
Area of an Isosceles Triangle
To determine the area of an isosceles triangle, you’ll need to know the length of its base and height. The formula for calculating the area is quite simple:
Area = (base × height) ÷ 2
Keep in mind that you might need to use the Pythagorean theorem or other geometric principles to find the height of the triangle in certain cases.
Perimeter of an Isosceles Triangle
Calculating the perimeter of an isosceles triangle involves adding the lengths of all three sides. Since the triangle has two equal sides, the formula for the perimeter can be expressed as:
Perimeter = base + 2 × (length of equal sides)
By mastering these calculations, you’ll be well-equipped to explore the fascinating world of isosceles triangles and the countless geometric wonders they encompass.
Isosceles Triangle Examples: Bringing Concepts to Life
To truly grasp the unique properties and characteristics of isosceles triangles, it’s important to dive into some real-life examples. These practical scenarios will reinforce your understanding of isosceles triangles and demonstrate how their properties can be applied in various mathematical contexts.
Example 1: Perimeter of an Isosceles Triangle
Consider an isosceles triangle with a base measuring 6 cm and equal sides measuring 8 cm each. To find the perimeter, we’ll use the formula:
Perimeter = base + 2 × (length of equal sides)
Plugging in the values for the base and equal sides, we get:
Perimeter = 6 + 2 × 8 = 6 + 16 = 22 cm
In this example, the perimeter of the isosceles triangle is 22 cm.
Example 2: Vertex Angle of an Isosceles Triangle
Suppose we have an isosceles triangle with base angles measuring 45 degrees each. To find the vertex angle, we’ll use the fact that the sum of all three angles in a triangle is always 180 degrees. Let’s denote the vertex angle as “V”:
Base angles + Vertex angle = 180 degrees
Since both base angles are equal, we can simplify the equation as:
2 × 45 degrees + V = 180 degrees
Solving for V, we get:
V = 180 degrees – 90 degrees = 90 degrees
In this example, the vertex angle of the isosceles triangle is 90 degrees.
These examples showcase the diverse ways in which the properties of isosceles triangles can be utilized to solve mathematical problems. By working through various examples and scenarios, you’ll develop a deeper appreciation for the elegance and versatility of isosceles triangles in the world of geometry.
Conclusion
As we conclude our exciting adventure into the captivating world of isosceles triangles, we can’t help but marvel at the diverse and fascinating properties these geometric wonders possess. From understanding their unique characteristics to exploring their real-world applications, we’ve embarked on a remarkable learning journey together, made even more delightful by the engaging and interactive approach of Brighterly.
At Brighterly, our mission is to ignite a passion for mathematics in children of all ages, fostering a love for learning that transcends traditional boundaries. By delving into the wonders of isosceles triangles, we’ve unlocked new doors to mathematical exploration, reinforcing the idea that math can be fun, accessible, and enjoyable for everyone.
Frequently Asked Questions on Isosceles Triangle
Can an isosceles triangle be a right triangle?
Yes, an isosceles triangle can be a right triangle. In this case, the triangle is called an isosceles right triangle. It has a 90-degree vertex angle, and the two equal sides are also the legs of the right triangle.
Can an isosceles triangle be an equilateral triangle?
An equilateral triangle is a special case of an isosceles triangle where all three sides are of equal length. So, technically, an equilateral triangle can be considered an isosceles triangle.
How do I find the height of an isosceles triangle?
To find the height of an isosceles triangle, you can use the Pythagorean theorem. The height divides the base into two equal parts, creating two right triangles. In each right triangle, the height is one leg, half of the base is the other leg, and the equal side of the isosceles triangle is the hypotenuse. Then, use the Pythagorean theorem to solve for the height.
Information Sources
For more information about isosceles triangles, you can visit the following websites:
- Wikipedia – Isosceles Triangle
- Math is Fun – Isosceles Triangles
- MathWorld – Isosceles Triangle
- National Council of Teachers of Mathematics – Isosceles Triangles
- Geogebra – Isosceles Triangle Activities
Keep practicing and exploring the fascinating world of triangles, and don’t forget to have fun along the way! Stay tuned for more exciting math topics on Brighterly.