Right Triangle – Definition with Examples
11 minutes read Triangles – they’re everywhere! Look around, and you might notice them in everyday objects or structures, like the roof of a house, the sail of a boat, or even a slice of your favorite pizza. But, have you ever considered what makes a triangle a right angled triangle?
At Brighterly, we love making math enjoyable and easy to grasp. So let’s embark on an exciting journey of discovery into the magical world of right angled triangles! You’ll find these triangles are not just fascinating shapes; they’re also crucial in understanding many concepts in geometry and beyond.
Did you know the great Pyramids of Egypt, one of the seven wonders of the world, are gigantic examples of right angled triangles? Or that when you set a ladder against a wall, you’re creating a real-life example of a right angled triangle? Amazing, isn’t it?
Right angled triangles are all around us, in architecture, nature, and even the screen you’re reading this on. They’re fundamental shapes in the study of mathematics and physics. As we delve deeper into the properties, formulas, and types of right angled triangles, you’ll begin to see them everywhere and appreciate the beauty of this simple yet powerful shape!
What is a Right Triangle?
A right triangle, or a right-angled triangle, as the name suggests, is a special kind of triangle that carries a distinct feature – one of its internal angles measures exactly 90 degrees. This specific angle is referred to as the right angle. The concept of a right triangle is not just an abstract mathematical concept. It’s a fundamental shape that you can spot all around you, in architecture, art, and nature. When you think of a right triangle, imagine a corner of a book, the shapes on a chessboard, or a slope on a mountain! They’re everywhere once you start looking.
Features of a Right Triangle
A right triangle is not just any triangle. It has unique characteristics that set it apart:
-
Right Angle: The standout feature of a right triangle is its right angle. This angle, which measures precisely 90 degrees, is a cornerstone in geometry.
-
Hypotenuse: The hypotenuse is the longest side of the right triangle and is always located directly across from the right angle. Think of it as the ‘ruler’ of the triangle, as it’s the side that determines the size of the triangle.
-
Legs: The remaining two sides of the triangle are referred to as the legs. These are the sides that embrace the right angle and play a vital role in the Pythagorean theorem, which we’ll discuss next.
For even more detailed answers to your questions and a host of engaging practice problems, check out our Right Triangle Practice Worksheet on Brighterly. Dive deeper into the world of right-angled triangles, master the Pythagorean theorem, and explore the properties and types of right triangles. Practice makes perfect, and with Brighterly, math has never been more fun and accessible!
Right Triangle Formula
Perimeter of a Right Triangle
The perimeter of a right triangle is the total distance around its edges. It’s like taking a walk along the triangle’s boundary! To calculate the perimeter, you simply add up the lengths of all three sides:
Perimeter = length of hypotenuse + length of leg1 + length of leg2
Pythagoras Theorem
The Pythagorean theorem is a fundamental principle in geometry, named after the ancient Greek mathematician Pythagoras.
Hypotenuse² = Leg1² + Leg2²
The theorem states that in a right triangle, the square of the length of the hypotenuse (remember, that’s the longest side) is equal to the sum of the squares of the lengths of the other two sides, or legs. This relationship holds true for all right triangles, and it’s a wonderful demonstration of the harmony and balance within geometry.
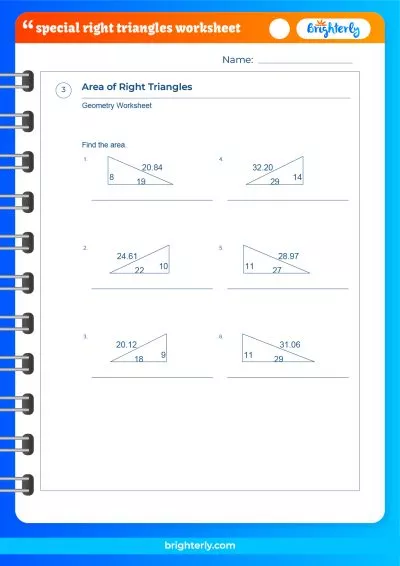
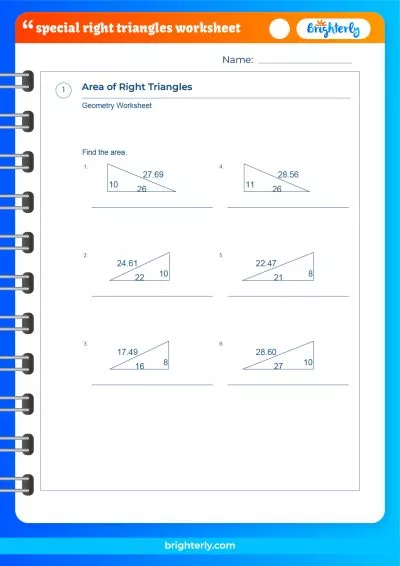
Right Triangle Area
Finding the area of a right triangle is like figuring out how much paint you’d need to cover its surface completely! The formula for the area is quite simple:
Area = 1/2 x base x height
In this equation, the base and the height represent the lengths of the two legs. It’s interesting to note that the hypotenuse does not factor into the area at all!
Properties of Right Angled Triangle
A right-angled triangle isn’t just unique because of its right angle. It has several other interesting properties:
-
One angle is always a right angle (90 degrees): This is the defining characteristic of a right triangle and the source of its name.
-
The sides of the triangle satisfy the Pythagorean theorem: This is a fundamental rule for right triangles. The squares of the lengths of the two legs add up to the square of the hypotenuse.
-
The hypotenuse is the longest side: No matter how the lengths of the legs change, the hypotenuse remains the longest side.
Types of Right Triangles
There are as many types of right triangles as there are stars in the sky! However, we will focus on two primary types:
Isosceles Right Triangle
An isosceles right triangle is a triangle in which the two legs are exactly the same length. This symmetry leads to some interesting properties: the two non-right angles are each 45 degrees, making it a perfect blend of equality and right angles!
Scalene Right Triangle
A scalene right triangle, on the other hand, is a bit more varied. In this triangle, all sides are of different lengths, and the non-right angles can be anything but 45 degrees. Often, these triangles have angles of 30 degrees and 60 degrees, but they can vary widely.
Practice Questions on Right Triangles
By now, you’ve gained a lot of knowledge about right triangles. But as with any new skill, practice makes perfect! Spend some time trying to solve problems involving right triangles. Draw them, measure them, calculate their area and perimeter, and use the Pythagorean theorem. The more you work with right triangles, the more comfortable you’ll become with them. Before long, you’ll be a right triangle expert, ready to tackle more complex mathematical concepts!
Conclusion
And just like that, we’ve journeyed through the world of right angled triangles together!
Understanding right triangles is more than just knowing their features or being able to use their formulas. It’s about appreciating the role these triangles play in our everyday lives and the world around us.
At Brighterly, we believe that everyone can master math when it’s presented in a fun, engaging, and relevant way. We hope this guide has given you a new perspective on right angled triangles. Remember, these triangles are not just shapes on a piece of paper; they’re the building blocks of the Pyramids, the foundation of trigonometry, and even the secret behind the screen you’re reading this on!
So keep exploring, keep asking questions, and keep practicing! After all, mathematics is an adventure, and you’re just getting started. Right angled triangles are just one fascinating stop on this journey.
We hope you continue to explore the world of mathematics with Brighterly. Remember, in the world of math, every question you ask, every problem you solve, brings you one step closer to becoming a true Math Master. And we’re here to help you every step of the way!
Frequently Asked Questions on Right Angle Triangle
What is a right triangle?
A right triangle, or a right-angled triangle, is a type of triangle that has one angle measuring exactly 90 degrees. The side opposite this right angle is the longest side and is called the hypotenuse. The other two sides are called legs. The right triangle is a fundamental shape in geometry and is used in many areas of mathematics and physics.
What is the Pythagorean theorem?
The Pythagorean theorem is a fundamental principle in geometry, named after the ancient Greek mathematician Pythagoras. It states that in any right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This theorem is a key to many mathematical problems and is fundamental in trigonometry.
What are the properties of a right triangle?
The key properties of a right triangle are: one angle is always a right angle (90 degrees); the sides of the triangle satisfy the Pythagorean theorem; and the hypotenuse is the longest side. These properties hold true for all right triangles and form the basis for many mathematical proofs and calculations.
What are the types of right triangles?
The two main types of right triangles are isosceles and scalene. An isosceles right triangle has two sides of equal length, which are the legs of the triangle. The angles of an isosceles right triangle are 45 degrees, 45 degrees, and 90 degrees. A scalene right triangle, on the other hand, has all sides of different lengths, and the angles can vary, typically being 30 degrees, 60 degrees, and 90 degrees.
How do you calculate the area and perimeter of a right triangle?
The area of a right triangle is calculated using the formula: Area = 1/2 x base x height, where the base and height are the lengths of the two legs. The perimeter of a right triangle is calculated by adding the lengths of all three sides:
Perimeter = length of hypotenuse + length of leg1 + length of leg2.
Sources
The information provided in this article is based on reputable sources from educational and government websites. Here are our references:
- Britannica – Pythagorean Theorem: Provides a deeper understanding of the Pythagorean theorem used in right triangles.
- Math Open Ref – Isosceles Right Triangle: Detailed information on the properties of an isosceles right triangle.
- Math Warehouse – Right Triangle FAQ: A list of frequently asked questions about right triangles to strengthen your understanding.
Remember, practice is key in mastering mathematical concepts. So, keep exploring, keep practicing, and you’ll find that math is not just about numbers, it’s also about patterns, shapes, and fascinating concepts like the right triangle!
Struggling with Geometry?
- Does your child struggle to grasp the basics of geometry?
- Try learning with an online tutor.
Kid’s grade
Is your child having difficulties with understanding geometry lessons? An online tutor could provide the necessary help.
Book a Free Class
