• Math tutors
• /
• Knowledge Base
• /
• Square – Definition, Properties, Examples, Facts

Square – Definition, Properties, Examples, Facts

Welcome to Brighterly, where we strive to make learning mathematics an enjoyable and engaging experience for kids! Our goal is to spark curiosity and inspire young minds to explore the wonders of mathematics. Today, we’re delving into the captivating world of squares. These fascinating shapes are not only essential in geometry, but they also have numerous practical applications in art, architecture, and design. So, without further ado, let’s embark on our journey to discover the amazing properties of squares!

What is a Square?

A square is a special type of quadrilateral, a two-dimensional shape with four sides and four angles. All four sides of a square are equal in length, and all four angles measure exactly 90 degrees. In other words, a square is a regular polygon with four equal sides and four equal angles. Squares are widely recognized and used in mathematics, art, and architecture, and they are essential building blocks in geometry.

Properties of a Square

Let’s discuss some of the unique characteristics that make squares stand out from other quadrilaterals:

1. All four sides are equal in length: A square is defined by having all its sides of equal length.
2. All four angles are right angles: Each angle in a square measures exactly 90 degrees.
3. Opposite sides are parallel: In a square, each pair of opposite sides is parallel to each other.
4. Diagonals are equal and bisect each other: A square has two diagonals that are equal in length and intersect each other at a 90-degree angle. These diagonals also bisect each other, meaning they divide each other into two equal parts.

Common Properties of a Square and Rectangle

Squares share some common properties with another popular quadrilateral, the rectangle. Both shapes have:

1. Four right angles: Both squares and rectangles have four 90-degree angles.
2. Opposite sides parallel: In both shapes, opposite sides are parallel to each other.
3. Diagonals bisect each other: In squares and rectangles, the diagonals intersect each other at their midpoints.

Formulas of a Square

In this section, we’ll explore some essential formulas related to squares:

1. Area: The area of a square can be calculated using the formula: Area = side × side or Area = side².
2. Perimeter: The perimeter of a square is calculated by adding the lengths of all four sides or using the formula: Perimeter = 4 × side.

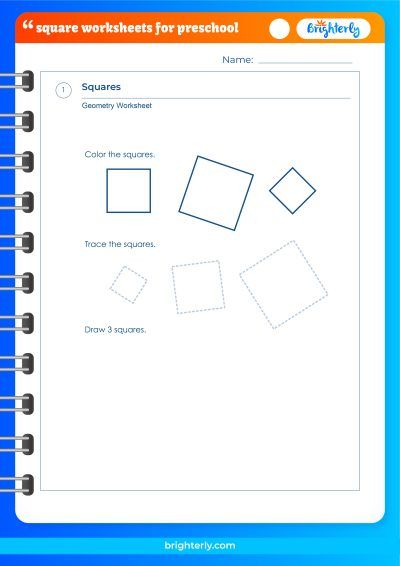
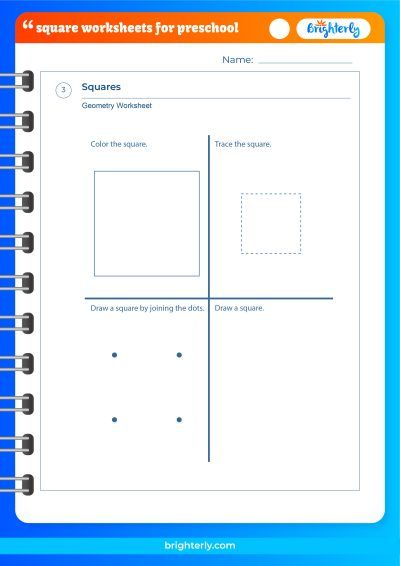
If you want to better master the topic of Square, we recommend that you pay attention to the math worksheets for kids from Brighterly. We will help you make learning math easy and fun.

Area and Perimeter of Square

Now that we know the formulas let’s understand how to calculate the area and perimeter of a square:

1. Area: To find the area of a square, simply multiply the length of one side by itself.
2. Perimeter: To calculate the perimeter of a square, multiply the length of one side by four.

Construction of a Square

To construct a square, follow these steps:

1. Draw a straight line segment of the desired length.
2. At each end of the line segment, draw a perpendicular line of equal length.
3. Connect the ends of the perpendicular lines to complete the square.

Diagonal of Square

The diagonal of a square can be found using the Pythagorean theorem. The formula to calculate the diagonal is: Diagonal = side × √2.

Solved Examples On Square

Let’s look at some solved examples to help you understand squares better:

Example 1: If the side of a square is 5 units, find its area and perimeter.

Solution:

• Area = side² = 5² = 25 square units
• Perimeter = 4 × side = 4 × 5 = 20 units

Example 2: If the diagonal of a square is 10 units, find its side length.

Solution:

• Diagonal = side × √2

• Side = Diagonal / √2 = 10 / √2 ≈ 7.07 units

Practice Problems On Square

Try solving these practice problems to sharpen your skills:

1. If the side of a square is 8 units, find its area and perimeter.
2. Calculate the length of the diagonal of a square with a side length of 6 units.
3. If the area of a square is 49 square units, find its side length and perimeter.

Conclusion

As we reach the end of our exploration, it’s clear that squares are not only fundamental shapes in geometry but also vital elements in various aspects of life. By learning about squares through the Brighterly platform, you’ve strengthened your understanding of mathematics and developed an appreciation for the beauty and practicality of geometric shapes. Whether you’re tackling complex mathematical problems or appreciating the symmetrical patterns in nature and architecture, your knowledge of squares will undoubtedly serve you well.

At Brighterly, we believe that the seeds of curiosity, creativity, and critical thinking are sown through engaging and enjoyable learning experiences. We hope that our journey through the realm of squares has been a rewarding and enriching adventure for you. Stay curious, keep exploring, and remember that the world of mathematics is vast and full of wonders waiting to be discovered!

Frequently Asked Questions On Square

What is the difference between a square and a rectangle?

Both squares and rectangles are quadrilaterals, which means they are two-dimensional shapes with four sides and four angles. They share some common properties, such as having four right angles (90 degrees) and parallel opposite sides. However, there is a key difference between the two shapes:

A square has all four sides equal in length, making it a regular polygon. In contrast, a rectangle has two pairs of equal sides, where the length of one pair is different from the other. This difference in side lengths distinguishes rectangles from squares.

How can I find the side length of a square if I know its area?

To determine the side length of a square when you know its area, you can use the formula for the area of a square, which is Area = side². To find the side length, you need to isolate the “side” variable by taking the square root of the area.

For example, if you know the area of a square is 36 square units, you can find the side length by calculating the square root of 36:

Side = √Area = √36 = 6 units

In this case, the side length of the square is 6 units.

What is the relationship between the diagonal and the side of a square?

The diagonal of a square has a specific relationship with the side length, which can be determined using the Pythagorean theorem. In a square, the diagonal divides the square into two right-angled triangles, where the diagonal acts as the hypotenuse and the two sides of the square act as the other two sides of the triangle.

The Pythagorean theorem states that the sum of the squares of the two shorter sides in a right-angled triangle equals the square of the hypotenuse. In the context of a square, the theorem can be written as:

Side² + Side² = Diagonal²

Since both sides are equal in length, you can simplify the equation to:

2 × Side² = Diagonal²

Solving for the diagonal, you get:

Diagonal = √(2 × Side²) = Side × √2

This formula shows the relationship between the diagonal and the side of a square, where the diagonal is equal to the side length multiplied by the square root of 2.

Information Sources

For further information, check out these reputable sources:

1. Square (geometry) – Wikipedia
2. Square – National Council of Educational Research and Training (NCERT)
3. The Geometry of the Square – Wolfram MathWorld