Subtract Fractions with Unlike Denominators – Definition with Examples
7 minutes read Welcome to Brighterly, where learning math is both fun and engaging. Today, we dive deep into a pivotal mathematical concept—Subtracting Fractions with Unlike Denominators.
Understanding fractions is a critical step in every child’s math journey. Subtracting fractions, particularly those with unlike denominators, might seem intimidating at first. But here at Brighterly, we believe in breaking down complex topics into easily digestible, comprehensible chunks. Armed with interactive examples and step-by-step guidance, your child will be subtracting fractions with unlike denominators with ease and confidence!
How to Subtract Fractions with Unlike Denominators
Understanding how to subtract fractions with unlike denominators is a key skill for young learners to develop. This essential competency often serves as a foundation for more complex math concepts that children encounter as they progress in their education. Before diving into the process, let’s clarify what it means to subtract fractions with unlike denominators.
What is Subtracting Fractions with Unlike Denominators?
In simple terms, subtracting fractions with unlike denominators refers to the process of subtracting two fractions where the bottom numbers (denominators) are not the same. Unlike adding and subtracting whole numbers, this requires an extra step to create equivalent fractions with a common denominator.
Steps For Subtracting Fractions with Unlike Denominators
Subtracting fractions with unlike denominators involves a four-step process:
- Identify the Least Common Denominator (LCD)
- Rewrite the fractions with the LCD
- Subtract the numerators
- Simplify the resulting fraction if possible
Children may find these steps challenging initially, but with enough practice, it becomes an easy task. It’s important to remember that math skills develop over time and practice is key.
Types of Fractions
Before proceeding to the methods of subtraction, it’s important to understand the types of fractions involved. Primarily, there are two types of fractions: proper fractions and improper fractions. A proper fraction is one where the numerator (top number) is less than the denominator, while an improper fraction has a numerator larger than or equal to the denominator. Knowing the types of fractions will help in understanding the subtraction methods better.
Methods to Subtract Fractions with Unlike Denominators
The subtraction of fractions with unlike denominators can be executed through several methods.
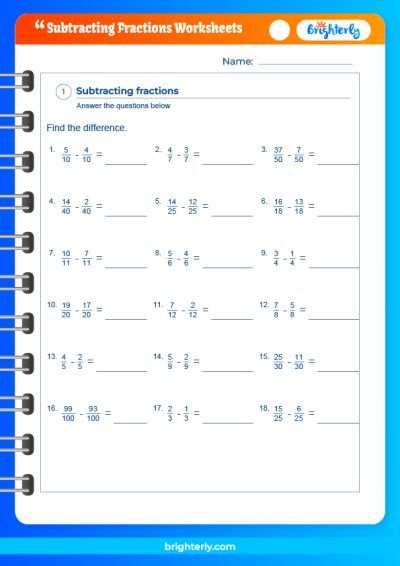
You can find the answers and additional practice questions in our Subtract Fractions Worksheets. This worksheet is designed to reinforce the concept of converting measurements from inches to centimeters.
Method I
This method involves finding the least common denominator (LCD), rewriting the fractions using this denominator, and then subtracting the numerators.
Method II
This method, also known as the Cross-Multiplication Method, eliminates the need to find the LCD but requires more multiplication steps.
Method III
This method is best used for mixed numbers (numbers with both a whole number and a fraction), as it involves first converting the mixed numbers to improper fractions.
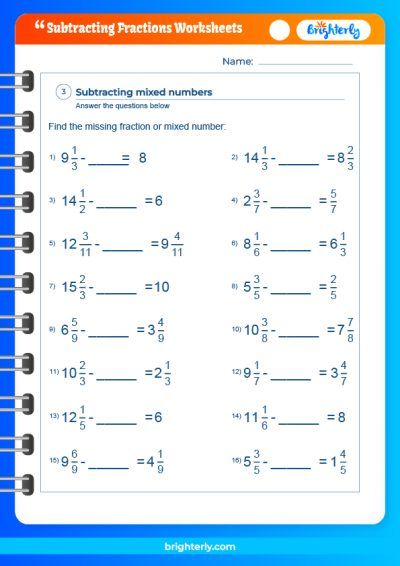
Subtracting Mixed Fractions with Unlike Denominators
Subtracting mixed fractions requires the same basic principles as subtracting proper fractions, but with an added step of dealing with the whole numbers. The key here is to handle the whole numbers and fractions separately.
Subtracting Fractions with Unlike Denominators Examples
To give you a better understanding of these concepts, we’ve put together a few examples. Remember, the key to mastering these concepts is through consistent practice!
Subtracting Fractions with Unlike Denominators Questions
To further reinforce these concepts, we’ve compiled a set of practice problems for your child. Encourage them to try these questions on their own to gain confidence and proficiency in subtracting fractions with unlike denominators.
Conclusion
In conclusion, subtracting fractions with unlike denominators is a crucial skill that forms the backbone of many advanced mathematical concepts. Here at Brighterly, we strive to make such foundational topics approachable and engaging for young learners.
By understanding and practicing the subtraction of fractions with unlike denominators, students not only enhance their mathematical abilities but also develop logical thinking and problem-solving skills. The process, which might seem intimidating initially, soon becomes an effortless task with regular practice and the right guidance.
FAQs on Subtracting Fractions with Unlike Denominators
What is the least common denominator (LCD) and how do I find it?
The least common denominator (LCD) is the smallest multiple that two or more denominators have in common. To find the LCD, follow these steps:
- Identify the denominators of the fractions you want to subtract.
- Determine the prime factors of each denominator.
- Take the highest power of each prime factor that appears in any denominator.
- Multiply all the prime factors obtained in the previous step to find the LCD.
For example, let’s say we want to subtract 1/3 from 2/5. The prime factors of 3 are 3, and the prime factors of 5 are 5. Since no prime factors are common, the LCD is simply 3 * 5 = 15.
Can I subtract fractions with different denominators without finding the LCD?
Yes, you can use alternative methods to subtract fractions with unlike denominators, such as the cross-multiplication method or converting the fractions to equivalent fractions with the same denominator. These methods eliminate the need to find the LCD. However, finding the LCD is often considered the most straightforward and efficient approach, especially when dealing with more complex fractions.
What should I do if the resulting fraction after subtraction cannot be simplified?
If the resulting fraction cannot be simplified further, it is already in its simplest form. This means that the numerator and denominator have no common factors other than 1. In such cases, the fraction is considered fully simplified.
Can I subtract mixed fractions with unlike denominators?
Yes, you can subtract mixed fractions with unlike denominators. To do so, follow these steps:
- Convert the mixed fractions into improper fractions.
- Find the LCD of the denominators.
- Rewrite the fractions with the LCD.
- Subtract the numerators.
- Simplify the resulting fraction, if possible.
- Convert the fraction back to a mixed fraction, if desired.
Information Sources
To ensure your child’s mathematical journey is well-supported, we recommend the following additional resources:
Struggling with Fractions?
- Does your child need additional support with understanding fractions lessons?
- Start lessons with an online tutor.
Kid’s grade
Is your child finding it challenging to master fractions concepts? An online tutor could be the answer.
Book a Free Class
