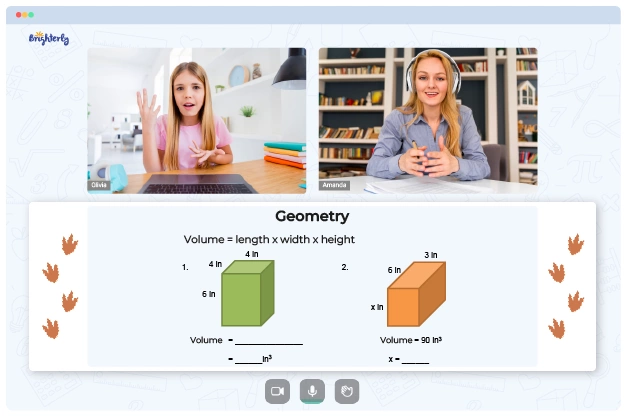
Volume of Triangular Pyramid
Updated on March 10, 2026
The volume of a triangular pyramid is found by multiplying one-third of the base area by the height. This article will explore all the nuances you need to know about the triangular pyramid volume, from its basic parts to the specific formulas you need, and practice with some fun exercises.
What is the volume of a triangular pyramid?
The volume of a triangular pyramid is the amount of inner space it contains. Think of it this way: if you were to empty a pyramid and fill it with water, the amount of water it could hold would be its exact volume. Mathematically, we measure volumes in cubic units, like cm^3, m^3, and so on.
An important thing about the triangular pyramid is the one-third rule. According to this rule, the triangular pyramid will have one-third of the volume of a prism with the same base surface area and height.
Triangular pyramid and its parts
A triangular pyramid is a polyhedron with the following characteristics:
- The base is the bottom face of the pyramid, which is always a triangle. This triangle can be equilateral, isosceles, or scalene.
- Apex is the topmost point (vertex) where all three lateral faces meet.
- Lateral faces are the three triangles that connect the base to the apex. Think of them as the pyramid sides.
- Height (h) is the perpendicular distance from the apex straight down to the center of the base. It must form a 90° angle with the base. It is also known as the altitude.
- The slant height (l) is the distance from the apex down the center of one of the lateral faces.
- Vertices and edges are the other parts. A triangular pyramid has 4 vertices (corners) and 6 edges.
It’s important that you do not confuse slant height with the actual height when calculating the volume of triangle pyramid!
Volume of a triangular pyramid formula
To calculate the volume of a pyramid with a triangular base, you need to know and use a formula:
V = (1/3) × B × h
The formula says that the volume (V) is exactly one-third the product of the base area (B) and altitude (a) of the bottom triangular face. Pretty simple, isn’t it? Once you understand how the formula works, you can treat it as a sort of volume of a triangular pyramid calculator: you substitute the letters with numbers.
How to find the volume of a triangular pyramid?
Now, let’s have a look at how exactly you can find the volume of a triangular pyramid step by step.
Since every pyramid is essentially one-third of a prism with the same dimensions, you just need to calculate the base area and the height to find the total space inside.
- The first step is finding the base. The base is a normal triangle; treat it as such. Multiply its base by its height and divide by 2.
- Next, identify the pyramid height, which is the vertical distance from the apex straight down to the base.
- Lastly, multiply your base area (B) by the height (h), and divide the final result by 3.
For example, if your base area is 30cm^2 and the pyramid is 10cm tall, you do a simple multiplication, like so:
30 x 10 = 300 cm^3
Then, divide the final volume by 3:
300/3 = 100 cm^3. So, the volume of a regular triangular pyramid at hand is 100 cm^3.
Always remember to express your final result in cubic units, so you show that you are measuring a three-dimensional space.
Facts about the volume of a triangular pyramid
A triangular pyramid is a very special shape. Beyond the basic calculations, they have several unique properties making them quite fascinating to study. Let’s look at some of those properties.
- One of the most important facts is that a triangular pyramid occupies exactly one-third of the volume of a triangular prism with the same base and height. In practice means that if you had a hollow prism and a pyramid of the same size, it would take exactly three full pyramids of water to fill the prism.
- The volume of a pyramid depends only on its base area and perpendicular height. Whether the pyramid is straight or tilted, the volume remains consistent as long as the base area and vertical height remain the same. This is known as Cavalieri’s Principle.
- Sometimes, the base and all three lateral sides are identical. In this case, we call these pyramids a regular tetrahedron. What’s so fascinating about this shape is that any of its four faces can serve as the base, and the volume calculation will always have the same result.
- On a more practical side, because of the triangular pyramid volume formula and how the volume is distributed toward the base, triangular pyramids are incredibly stable. They can support significant weight, and that’s why they are are commonly used in engineering and architecture.
Solved examples for the volume of a triangular pyramid
Solved example 1
A triangular pyramid has a base area of 24 cm² and a vertical height of 9 cm. What is its volume?
Solution: First, identify the values: B = 24, h = 9
Then, apply the formula for volume of a triangular pyramid: V = (1/3)Bh
Substitute the letters with the numbers to get V = (1/3) x(24×9)
The calculate, like so V = (1/3) x (216) = 72cm³
| The volume is 72 cm³. |
Solved example 2
Find the volume of a pyramid, the triangular base of which has a base length of 10 cm and an altitude of 6 cm. The pyramid itself is 12 cm tall.
Solution: First, find the base area of the triangle.
B = (1/2) x (base x height) = (1/2)(10 x 6) = 30 cm²
Next, apply the volume formula and add in the numbers:
V = (1/3)Bh = (1/3)(30×12) = 120 cm³
| The volume is 120 cm³. |
Solved example 3
The volume of a triangular pyramid is 90 cm³, and the base area is 15 cm². Find the height.
Solution: You need to start with the formula and substitute known values:
V = (1/3)Bh
90 = (1/3) x (15 x h)
Next, multiply both sides by 3 to get 270 = 15h
To get the height, divide both by 15:
h = 270/15 = 18
| The height of your pyramid is 18 cm. |
Practice problems on the volume of a triangular pyramid
Practice problem 1: A triangular pyramid has a base area (B) of 15 cm² and a vertical height of 6 cm. Calculate the pyramid’s volume.
Practice problem 2: Find the volume of a pyramid where the base length of the triangular base is 8 in, and the altitude is 3 in. The height of the pyramid is 10 in.
Practice problem 3: A regular tetrahedron has a base area of 21 m² and a height of 7 m. What’s the volume of the tetrahedron?
Frequently asked questions on the volume of a triangular pyramid
How to find the volume of a triangular pyramid?
Start by calculating the area of the triangular base first. Then multiply that base area by the pyramid’s height (the vertical distance from the apex to the base) and divide the final result by 3. Make sure you use the vertical height, not the slant height; it’s a common confusion.
What is the surface area and volume of a triangular pyramid?
The volume of a triangular pyramid measures the internal space of the pyramid (or how much water you could fill the pyramid with, if you emptied it out). The surface area, on the other hand, measures the total exterior coverage of the pyramid.
What is the formula for the volume of a triangular pyramid?
The formula for triangular pyramid volume is V = (1/3)Bh, where V is volume, B is base, and h is height. The formula says you multiply the base by the height of the pyramid, then divide the result by 3. The answer will be the volume of your pyramid.
Problems with Geometry?
- Does your child need additional assistance with grasping geometry basics?
- Start learning with an online tutor.
Choose kid's grade
Is your child finding it challenging to master geometry concepts? An online tutor could be the solution.
Book FREE lesson
