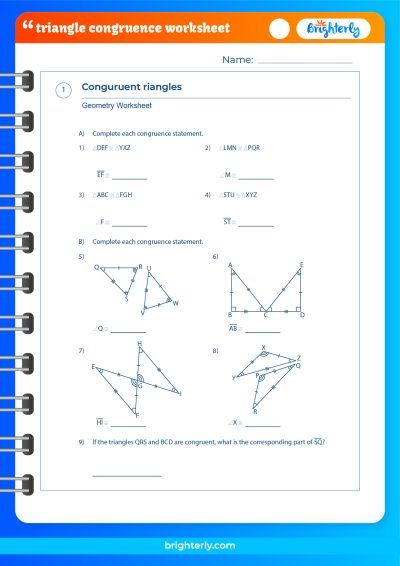
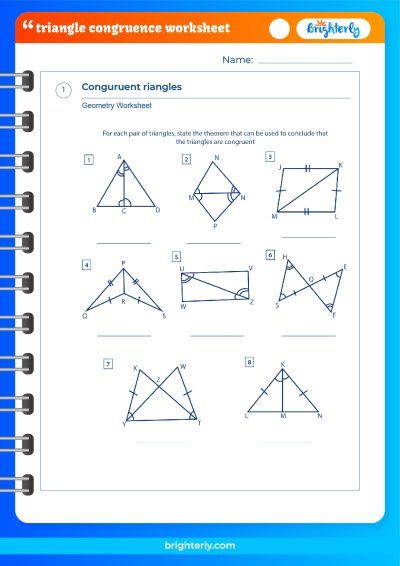
Congruent Angles – Theorem, Definition With Examples
Updated on January 9, 2024
At Brighterly, we consider mathematics to be a wonder because of its accuracy, simplicity, and ability to explain the reality in which we live. Today, one of the fundamental ideas of geometry—congruent angles—will be examined in depth.
What Are Congruent Angles?
Intriguing ideas that are fundamental to our comprehension of the cosmos are revealed by the interesting field of geometry. Congruent angles is one of these important ideas. Congruent angles are, to put it simply, angles with the same measure or degree.
Visualize this situation: In your hands are two mouthwatering pizza slices. One is from a smaller pie, the other from a larger one. Though the arcs and side lengths differ, both tips make a 30-degree point. Their angles match, so they’re congruent slices. Similar to how appetizing pizza may come in many sizes with identical angles, geometry offers an endless quantity of congruent angles in all shapes and sizes. Corners come together nicely, regardless of their size.
Congruent Angle Definition
Congruent angles are officially defined this way: two angles are congruent if their measures are identical. By this principle, congruence is irrespective of side lengths or dimensions.
Remember that congruent angles can take different positions or alignments. A 45-degree angle, for instance, may be inclined, upright, or flat. But as long as the measurement is 45 degrees, all variations remain congruent, regardless of orientation.
Congruent Angle Theory
According to the congruent angles postulate, also known as the theory of congruent angles, two angles are said to be congruent if their measures are equivalent. This idea is the foundation upon which many geometric arguments and constructions are based.
This merely restates the notion of congruent angles, providing a solid, unquestionable foundation for further geometric investigations. It makes the idea more solid, allowing pupils to understand increasingly difficult mathematical ideas. The theory gives us the fundamental knowledge that opens up new avenues for geometric discoveries. The theory of congruent angles provides a solid foundation for further geometric explorations by axiomatizing the concept.
Properties of Congruent Angles
The property is their independence from the size of the angle’s sides. Whether you have a broad, sweeping angle or a tight, narrow one, if the measures are identical, the angles are congruent.
Congruent angles, to sum up, always equal the same thing. Two angles are congruent to one another if they are both congruent to a third angle. Congruent angles have this characteristic, called transitivity, which makes them important.
At Brighterly, we think that mastery comes from practice. We thus encourage you to look through our congruent angles worksheets, which provide a variety of extra practice problems with solutions.
Practice Problems on Congruent Angles
Congruence of Complementary Angles:
If ∠L and ∠M are complementary, m∠L = 40°, are ∠L ≅ ∠M? Find m∠M.
Angle Measures of Congruent Supplementary Angles:
If supplementary ∠X ≅ ∠Y, find the measure of each.
Quadrilateral Angle Congruence:
In quadrilateral ABCD, ∠A ≅ ∠C and ∠B ≅ ∠D. If m∠A = 120° and m∠B = 85°, find m∠C and m∠D.
Frequently Asked Questions on Congruent Angles
What does it mean for angles to be congruent?
Two angles are said to be congruent when they have the exact same measure, regardless of their orientation or the lengths of their sides. It’s all about the degree measurement; if that’s the same, then the angles are congruent!
Can vertical angles be congruent?
Yes, vertical angles are always congruent. Vertical angles are the angles opposite each other when two lines cross. They are called “vertical” because they share the same vertex. According to the Vertical Angles Theorem, these angles are always congruent.
How do I write equations involving congruent angles?
When writing equations involving congruent angles, the congruence symbol (≅) is used. If you know that angle A is congruent to angle B, you would write this as ∠A ≅ ∠B.
Information Sources
Homework help with math tutor [grade 1-9]
Help your child achieve their full math potential
Choose kid's grade
Math homework help for grade 1-9
Join Brighterly and boost your child's math skills with our best tutors.
