Dividing Mixed Numbers
Updated on January 5, 2024
Welcome to Brighterly, your mathematical compass guiding you through the labyrinth of mathematical concepts. Today, we are embarking on a journey through the captivating realm of fractions, specifically dividing mixed numbers. This might seem like a formidable expedition initially, but with the right knowledge and practice, it will turn into an enjoyable adventure. Let’s join forces, sharpen our mathematical swords, and conquer the dragon of mixed numbers division together!
Mixed numbers, a unique blend of whole numbers and fractions, have their own set of rules for mathematical operations. Whether it’s addition, subtraction, multiplication, or division, understanding these rules can make math considerably more manageable and even fun. So, tie your mathematical boots tightly, grab your numerical shield, and let’s step into the kingdom of mixed numbers.
Dividing Mixed Numbers – Introduction
Welcome to Brighterly, your beacon of knowledge in the vast sea of mathematical conundrums. Today, we’re setting sail into the mesmerizing world of fractions, zooming in on a special type of number known as mixed numbers. Navigating the waters of dividing mixed numbers can be a bit challenging, but don’t worry! Brighterly is here to chart your course and help you master this important skill. Before we start dividing mixed numbers, however, it’s crucial to understand what they are, and to get acquainted with a few key concepts. So, let’s hoist the sails and embark on this educational voyage together!
What Are Mixed Numbers?
In the bustling town of numbers, mixed numbers are unique residents. A mixed number is a fascinating amalgamation of a whole number and a fraction. For instance, numbers like 2 1/2, 5 3/4, and 7 1/3 are all mixed numbers. Each one of these numbers is a blend of two parts: a whole number (like 2, 5, or 7) and a proper fraction (like 1/2, 3/4, or 1/3). This unique combination allows us to represent quantities that are more than a whole but less than the next whole number.
Proper Fractions
Proper fractions are humble, down-to-earth fractions where the numerator (the top number) is less than the denominator (the bottom number). They are called ‘proper’ because they properly fit within a whole. Examples of these are 1/2, 3/4, and 2/3, each representing a part of a whole that’s less than the whole itself.
Improper Fractions
Contrarily, improper fractions are those fractions where the numerator is equal to or greater than the denominator. These fractions aren’t improper because they’re incorrect, but because they represent a quantity greater than or equal to a whole. Examples include 3/3 (which equals to one whole), 4/2 (equals two wholes), or 5/4 (exceeds one whole).
Mixed Numbers
As we’ve seen, mixed numbers are an engaging fusion of whole numbers and proper fractions. This amalgamation allows us to express quantities more precisely than whole numbers alone and more intuitively than improper fractions. Mixed numbers can help us represent real-world quantities that exceed a whole but don’t reach the next whole number, like 1 1/2 pizzas or 2 3/4 hours.
Related Worksheets
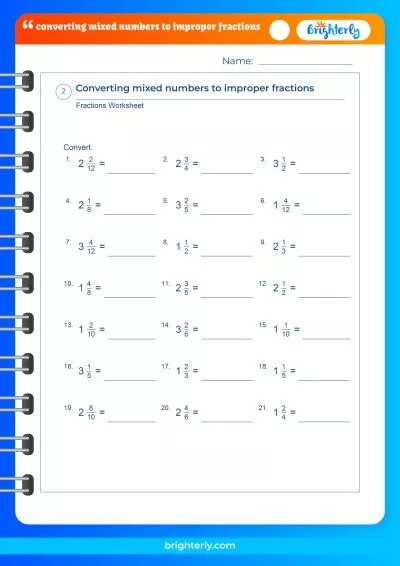
At Brighterly, we believe that practice is the key to mastering any mathematical concept. To aid your journey towards mastery, we’ve prepared some exciting related worksheets. These worksheets are designed to give you ample opportunities to practice converting and dividing mixed numbers. You can find these learning treasures in the worksheets section on our website.
How to Convert Mixed Numbers into Improper Fractions?
When it comes to dividing mixed numbers, our first step is to convert them into improper fractions. It might sound like a daunting task, but it’s as simple as a sailor tying a knot! All you need to do is multiply the whole number by the denominator of the fraction, then add the numerator. The result becomes the new numerator of your improper fraction, while the denominator remains unchanged.
How to Divide Fractions?
Now that we’re equipped with our improper fractions, the next step in our journey is to learn how to divide fractions. Here’s your map: Keep the first fraction as it is, change the division sign to multiplication (this is known as taking the reciprocal), and flip the second fraction. Then, multiply the numerators together to get the new numerator, and do the same with the denominators for the new denominator. If possible, simplify your answer to its lowest terms.
How to Divide Mixed Numbers?
With the skills of converting mixed numbers into improper fractions and dividing fractions in your mathematical toolkit, you’re now ready to tackle dividing mixed numbers. The process is just the same as the steps we’ve learned! Convert the mixed numbers into improper fractions, then follow the steps for dividing fractions.
Dividing a Mixed Number by Another Mixed Number
When you’re dividing a mixed number by another mixed number, it’s like having a friendly duel in the world of fractions. Convert both mixed numbers into improper fractions, and then divide the fractions as you normally would. Remember the rule – keep, change, flip, and you’re good to go.
Dividing Mixed Numbers by Fractions
If you’re dividing mixed numbers by fractions, treat it like a dialogue between a mixed number and a fraction. First, convert the mixed number into an improper fraction. Then, divide the improper fraction by the given fraction. Remember, the fraction is just another sailor in the sea of numbers, and our rules remain the same.
Dividing Mixed Numbers by Whole Numbers
When it comes to dividing mixed numbers by whole numbers, it’s like a conversation between a mixed number and a whole number. Here, convert the mixed number into an improper fraction. Then, think of the whole number as a fraction with the number as the numerator and 1 as the denominator. Follow the same steps as fraction division.
How to Divide Fractions with Mixed Numbers?
If you’re looking to divide fractions with mixed numbers, the process remains the same. Convert the mixed number into an improper fraction first. Then, proceed with the usual division process.
Solved Examples on Dividing Mixed Numbers
To bring these concepts to life, we’ve prepared some solved examples. These examples provide step-by-step solutions to various problems involving dividing mixed numbers. They are like guided tours through the process, showcasing how to apply the steps we’ve learned.
Practice Problems on Dividing Mixed Numbers
Practice makes perfect! To help you perfect your skills, we’ve included some practice problems on dividing mixed numbers. Remember, the more problems you solve, the better you’ll understand the process. So, roll up your sleeves and dive in!
Each of these sections builds upon the previous, leading you towards a comprehensive understanding of how to divide mixed numbers. Remember, at Brighterly, we’re always here to illuminate your learning journey. Happy exploring!
Conclusion
As we reach the end of our adventure through the land of mixed numbers division, we hope you’ve enjoyed the journey as much as we have. Here at Brighterly, we are committed to making math accessible, understandable, and enjoyable. Dividing mixed numbers might seem like scaling a mathematical mountain at first, but with the right tools and techniques, you’ll soon be standing atop, enjoying the view.
Remember, every mixed number division quest begins with converting mixed numbers into improper fractions. Once you have your improper fractions, the division becomes a straightforward path. It’s a journey of understanding, practice, and perseverance. As you continue exploring the mathematical world with us at Brighterly, you’ll find that no mountain is too high, no fraction too complex. Keep practicing, keep asking questions, and keep exploring!
Frequently Asked Questions on Dividing Mixed Numbers
In this section, we delve into the treasure trove of queries that we often encounter about dividing mixed numbers. Here, we aim to quench your thirst for knowledge, clearing any doubts that might be clouding your understanding.
What is the process of converting a mixed number into an improper fraction?
Converting a mixed number to an improper fraction involves a simple three-step process. First, multiply the whole number by the denominator of the fraction. Second, add the numerator to the product obtained in the first step. This sum becomes the new numerator of your improper fraction. Lastly, keep the denominator the same.
How do I divide mixed numbers?
When you’re tasked with dividing mixed numbers, start by transforming them into improper fractions. Once you’ve done that, remember the golden rule of fraction division – keep, change, flip. Keep the first fraction as it is, change the division operation to multiplication, and flip (find the reciprocal of) the second fraction. Multiply the numerators together for the new numerator, and do the same with the denominators for the new denominator. Simplify if possible.
What if I have to divide a mixed number by a whole number?
If you’re dividing a mixed number by a whole number, start as always by converting the mixed number into an improper fraction. Then, treat the whole number as a fraction with the number as the numerator and 1 as the denominator. Follow the usual rules of fraction division from there.
Information Sources:
Need help with Numbers?
- Is your child finding it hard to understand numbers fundamentals?
- An online tutor could be of help.
Choose kid's grade
Does your child struggle with mastering the basics of numbers? Try studying with an online tutor.
