Transitive Property – Definition with Examples
reviewed by Jo-ann Caballes
Updated on November 27, 2024
Welcome to Brighterly, where we make even the most complex-seeming math concepts easy to learn and fun to engage with!
Today, we’re going to be diving into the world of algebra and equalities and exploring the concept of transitive property.
Transitive property can help us apply logic to solve a range of math problems, including algebraic problems.
Here, we’ll find out the transitive property meaning, including the definition of the transitive property of equality, inequality and congruence, and the transitive property formula. We’ll also give you the tools you need to improve your knowledge further with practice test problems and math worksheets.
What is transitive property?
We define transitive property as a mathematical statement that can be applied to numbers, variables, algebraic expressions and even geometry. It states that if two entities are equal to the same entity, then they are also equal to one another. We cover the full transitive property definition below.
Transitive property: definition
The transitive property definition is a statement that represents how entities are related to one another. Usually, these entities will be represented by a, b and c. If a is equal to b, and b is equal to c, this must mean that a is equal to c. However, if a is larger than b, and b is larger than c, then a must be larger than c. This is an example of a transitive property equation.
Transitive property can be applied to equations, algebraic expressions, measurement conversions, geometrical shapes and line segments – for example, transitive property geometry can reflect if lines are parallel or congruent to one another.
Transitive property example
Here are some transitive property examples in action.
- x = y, and y = 9. This must mean that x = y.
- Line a is parallel to line b, and line b is parallel to line c. This means that line a and line c are also parallel.
- Danny is taller than Laura, who is taller than Lucas. This means that Danny is also taller than Lucas.
- If 36 inches is equal to 1 yard, and 1 yard is equal to 3 feet, this means that 36 inches is equal to 3 feet.
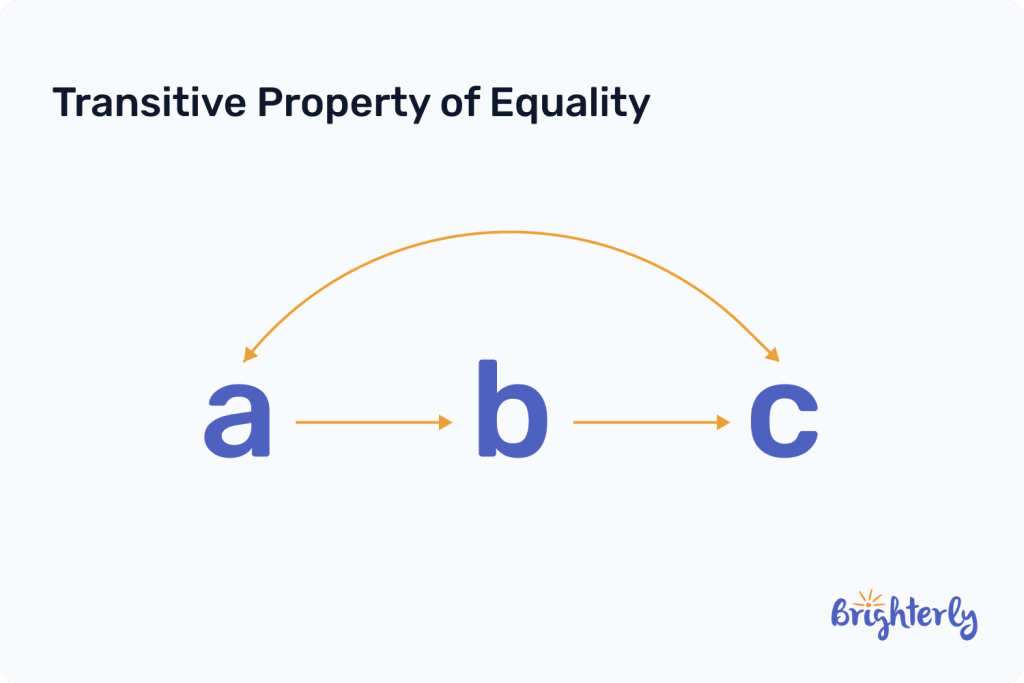
What is the transitive property of equality?
The transitive property of equality definition shows how properties are equal to one another. It states that if one property, for example, a, is equal to b, and b is equal to c, this means that a is also equal to c. A transitive property of equality example is: if a = b and b = c, then a = c too.
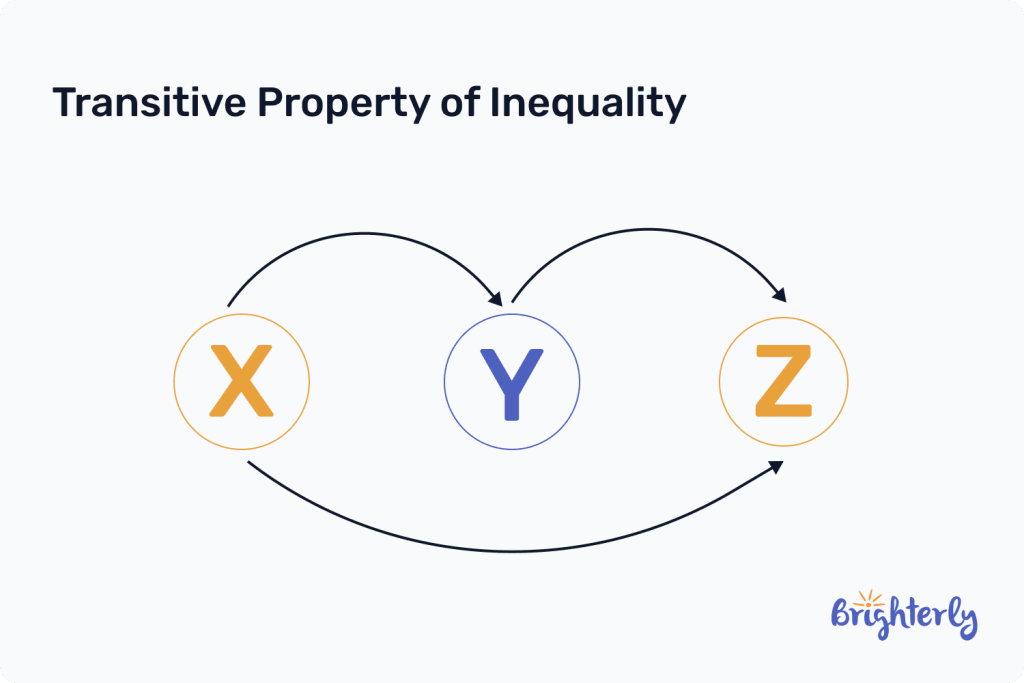
Transitive property of inequality
The transitive property of inequality represents the inequalities between different entities. Essentially, if a is larger than b, and be is larger than c, then we can confidently state that a is also larger than c. Similarly, if a is smaller than b, and b is smaller than c, then it must be true that a is smaller than c.
Transitive property of congruence
The transitive property of congruence describes how shapes are related to one another. We provide the full definition of the transitive property of congruence below.
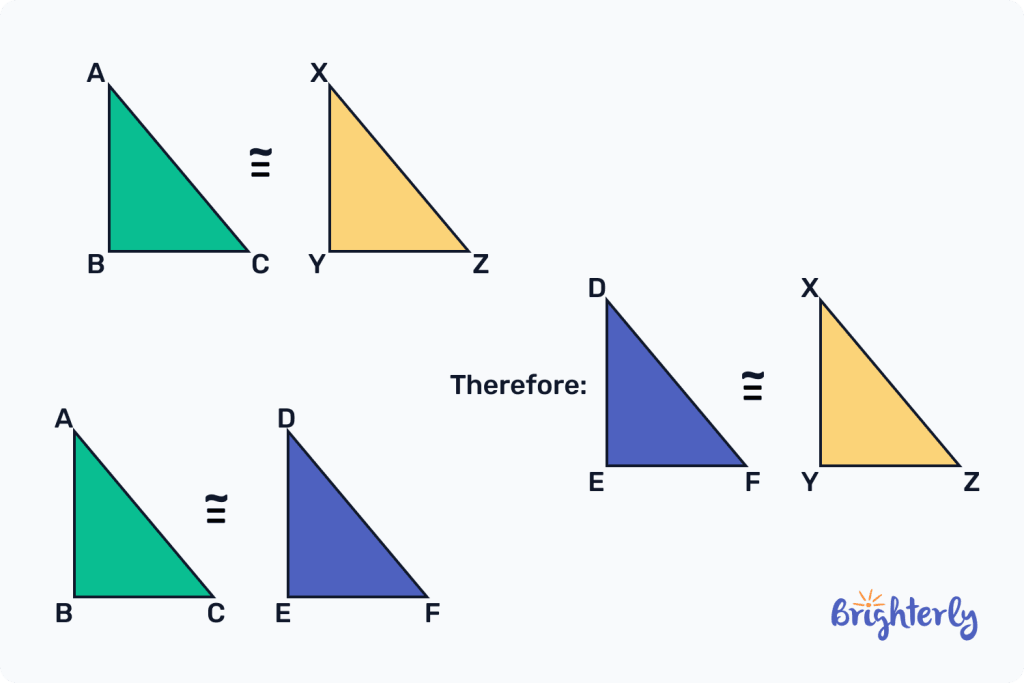
What is the transitive property of congruence?
The transitive property of congruence is a statement that says if a shape has the same shape or size as a second shape, which then has the same shape and size as a third shape, the first and third shapes are also the same shape and size aka congruent.
Put more simply, if triangle 1 is congruent to triangle 2, and triangle 2 is congruent to triangle 3, then shapes 1 and 3 are also congruent. This is an example of the transitive property of triangle congruence.
Transitive property of congruence example
Here are some transitive property of congruence examples:
- If angle a is equal to angle b, and angle b is equal to angle c, then angle a is equal to angle c
- If circle a is congruent to circle b, which is also congruent to circle c, then circle a and circle c are also congruent to one another
- If square a has side lengths of 5cm and is congruent to square b, which is also congruent to square c, we can say that squares a and c are also congruent and both have side lengths of 5cm
Transitive property formula
The transitive property formula is as follows:
If a = b, b = c, then a = c
When do we use transitive property?
We can use transitive property whenever we need to connect three entities together that are related by a rule. Here are some scenarios when we use transitive property:
- Transitive property of algebraic expressions
- Transitive property of angles
- Transitive property of congruence in parallel lines
Transitive property of algebraic expressions
We use the transitive property when showing the relationship between three algebraic expressions. This can be both the transitive property of equality and the transitive property of inequality.
For example, if we say x = y and y = z, this must mean that x = z. However, if we say x > y, and y > z, this must mean that x > z.
Transitive property angles
We can use transitive property to show the relationship between three related angles. For example, if angle a is congruent to angle b, and angle b is congruent to angle c, then angles a and c are also congruent. This is a transitive property of angle congruence.
Transitive property of congruence in parallel lines
The transitive property of congruence in parallel lines shows that three lines are parallel to one another. For example, if line x is parallel to line y, and line y is parallel to line z, we know that line x is also parallel to line z.
Solved math tasks: examples
Are you ready to put your knowledge of transitive property to the test? Try out our solved math problems – work out the answer yourself then check back in to see if you got it right. How many answers can you get right the first time?
Solved math task 1
What is the value of c if a = b, and b = 7?
Answer:
| 7. |
Through transitive property, we know that a and b are equal. Therefore, if b is 7, then a must also be 7.
Solved math task 2
What is the value of x if x = y, y = z and z = 3?
Answer:
| 3. |
Through the power of transitive property, we know if x and y are equal, and y and z are equal, then x and z are equal. This means that if z = 3, then x must also equal 3.
Solved math task 3
Which statement is an example of the transitive property of congruence?
Rectangle a = rectangle b, rectangle b = rectangle c, therefore rectangle a = rectangle c.
Or
Rectangle a = rectangle b, rectangle b < rectangle c, therefore rectangle a = rectangle c.
Answer:
| Rectangle a = rectangle b, rectangle b = rectangle c, therefore rectangle a = rectangle c is an example of the transitive property of congruence. |
This is because if rectangle a and b are equal, and rectangle b is smaller than rectangle c, rectangle a cannot be equal to rectangle c.
Transitive property: practice math problems
Transitive property: worksheets
Ready to take your knowledge of transitive property to the next level? Try out our fun, free math worksheets! They contain a range of puzzles and games that will not only improve your knowledge but will be exciting to work with!
- Equations and inequalities worksheets
- Evaluating expressions worksheets
- Algebraic expressions worksheets
- Parallel and perpendicular line worksheets
Online Winter Math Camp for Grades 1-8
Join us to help your kid achieve their full math potential.
Choose kid's grade
Online Winter Math Camp for Grades 1-8
Join our Math Winter Camp and boost your child's math skills with our best tutors.
