Value – Definition, Examples, Charts
reviewed by Jo-ann Caballes
Updated on December 26, 2024
When we hear value, we think of the worth of something but what does value mean in math? And, what’s the value of a number?
The value meaning in math is the same as the original concept of worth. However, this worth refers to that of a number or mathematical expression.
Here, we define value in math and explain how to find the value of a number through examples and practice problems.
What is Value?
Value is the significance, worth, or utility of something.
Value in math definition
Value definition in math is the worth of an expression, symbol, or number. This is its numerical quantity and it may be derived through calculation. For instance, if X = 2 + 4, then the value of X is 6.

How to find the value
Calculation may reveal the value of numbers, mathematical expressions, and problems, especially when they are unknown.
However, at times the value in math can be arrived at by observation. For instance, in the number 5,246, the value of 4 is 40. Another helpful way to find the value is by multiplying the face value by the place value.
Place Value
Place value is the value of a digit as determined by its position in a number. In our previous example of 5,246, the place value of 5 is thousands, 2 is hundreds, 4 is tens, and 6 is ones.

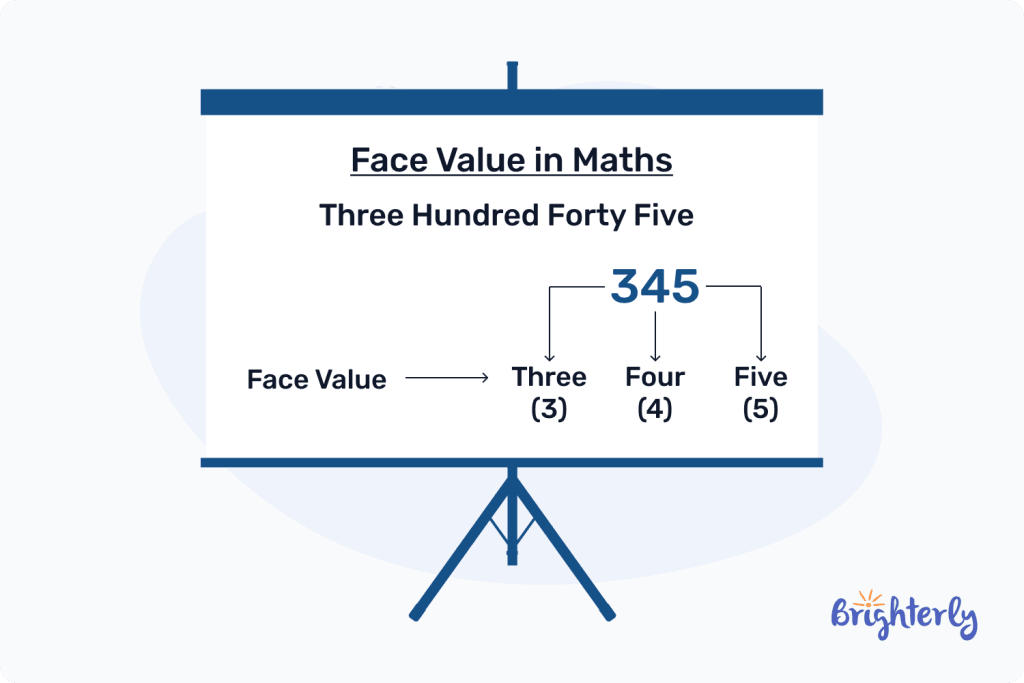
Face Value
The face value of a digit is what the digit is, regardless of its position in a number. The face value of 5 in 5,246 is 5.
Value
In a number, a digit’s value is the digit’s worth in that number. The worth of 6 in 623 is 600. The number value applies even when there is more than one of the same digit in a number. For instance, in 5,453, the value of the first 5 is 5,000 and the second 5 is 50. Remember that you can multiply the face value by the place value to know the value of a digit.
Value Table
A value table is a structured arrangement of numbers by putting each digit in a column and the place value, face value, and value are arranged in other columns. This way we can find the value of each digit by tracing the rows they fall into. For instance, if we want to know what value does each digit represent in the number 9,653,822, here’s how it would look:
| Digit | Place value | Face value | Value |
| 9 | Millions (1,000,000) | 9 | Nine million (9,000,000) |
| 6 | Hundred thousands (100,000) | 6 | Six hundred thousand (600,000) |
| 5 | Ten thousands (10,000) | 5 | Fifty thousand (50,000) |
| 3 | Thousands (1,000) | 3 | Three thousand (3,000) |
| 8 | Hundreds (100) | 8 | Eight hundred (800) |
| 2 | Tens (10) | 2 | Twenty (20) |
| 2 | Ones (1) | 2 | Two (2) |
Examples on Value
With the table given, we can find the value, place value, and face value of digits in numbers by substituting the figures for any number given. Let’s look at the value math example below:
What is the value of each number in 6,783?
| Digit | Place value | Face value | Value |
| 6 | Thousands (1,000) | 6 | Six thousand (6,000) |
| 7 | Hundreds (100) | 7 | Seven hundred (700) |
| 8 | Tens (10) | 8 | Eighty (80) |
| 3 | Ones (1) | 3 | Three (3) |
Now, we can identify the face value, place value, and value of each digit in the number.
Practice Questions on Value
Solved math problem 1
What is the value of 6 in the number 4, 363?
Solution
| The value of 6 in 4,363 is 60 (sixty). |
We can determine this by multiplying the face value (6) by its place value (10 or tens). 6 × 10 = 60
Solved math problem 2
What is the value of 4 in 9,321,644?
Solution
Again, when we multiply their place values by their face values, we can get their values.
4 × 10 = 40
4 × 1 = 4
| The value of the first 4 is 40 and the second 4 is 4. |
Value: practice math problems
Frequently Asked Questions on Value
What is the difference between place value and face value?
Place value is the value of a digit in a number as determined by its position while face value is what the digit is ordinarily. For 36, the place value of 3 is tens and the face value is 3.
How is value used in mathematics?
Value is used in mathematics to know the worth of a symbol, digit, or expression.
What is a value table?
A value table is a structured arrangement of numbers by putting each digit in a column and the place value, face value, and value are arranged in other columns.