Adding Exponents – Steps, Definition with Examples
Updated on January 5, 2024
Welcome to another exciting journey into the world of mathematics with Brighterly. Today, we are going to explore an essential mathematical concept that often appears in elementary arithmetic, algebra, and further advanced studies – the concept of exponents. By the end of this exploration, we aim to make you comfortable with the idea of adding exponents, explain the steps involved, and illustrate the process with easy-to-understand examples.
Whether you’re an ambitious student looking to stay ahead of your class, a dedicated parent hoping to support your child’s learning journey, or a teacher seeking to find interesting ways to teach this topic, we believe this guide will be beneficial. So let’s dive in and demystify the process of adding exponents together!
What Are Exponents?
Exponents, often referred to as powers, represent a fundamental concept in mathematics, playing a crucial role in various mathematical operations and equations. At its core, an exponent refers to the number of times a particular number, known as the base, is used in a multiplication. For instance, in the equation 2³, ‘2’ is the base, and ‘3’ is the exponent, indicating that ‘2’ is multiplied by itself three times.
The beauty of exponents lies in their simplicity and compactness. They help us write large numbers or repeated multiplication more succinctly. Imagine writing 2 x 2 x 2 x 2 x 2. It’s quite a hassle, isn’t it? Exponents simplify it to 2⁵, making it easier to read and write. Now, as we move forward, let’s take a closer look at the rules of adding exponents.
Definition of Exponents
In the realm of mathematics, exponents are used to express the repeated multiplication of a number by itself. The number that is being multiplied is referred to as the “base”, while the exponent denotes the number of times the base is multiplied. For example, in the expression 5⁴, ‘5’ is the base number, and ‘4’ is the exponent, suggesting that ‘5’ is multiplied by itself four times.
Adding Exponents – Definition and Process
Adding exponents doesn’t mean adding the exponents themselves. Instead, it implies multiplying the base numbers when the exponents and base numbers are the same. For instance, with 2³ + 2⁴, we don’t add 3 and 4, but we add the results of 2³ and 2⁴. Therefore, the primary operation in adding exponents is multiplication.
Properties of Exponents relevant to Addition
Understanding the properties of exponents is essential when dealing with exponent addition. There are four key properties to consider: the product of powers property (aᵐ.aⁿ = aᵐ⁺ⁿ), the quotient of powers property (aᵐ/aⁿ = aᵐ⁻ⁿ), the power of a power property ((aᵐ)ⁿ = aᵐ.ⁿ), and the zero exponent property (a⁰ = 1, for any a ≠ 0).
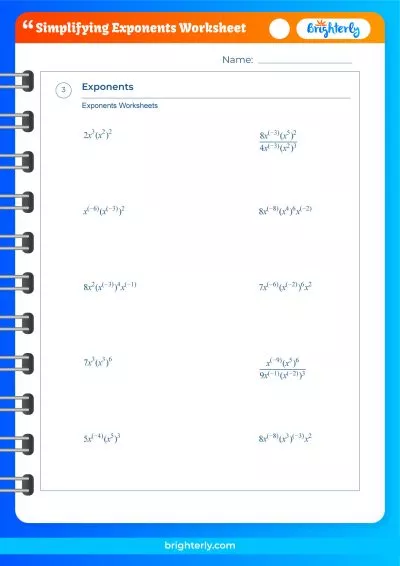
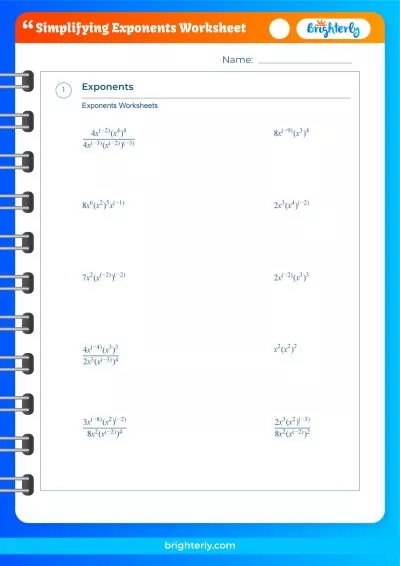
At Brighterly, we believe that practice is the key to mastery. That’s why we invite you to explore our adding exponents worksheets, where you can find an array of additional practice questions, complete with answers.
Properties of Base Numbers in Exponent Addition
The properties of base numbers play a significant role when adding exponents. If the base numbers are the same, the exponents can be added together. However, if the base numbers are different, the operations must be carried out separately before the results are added. It’s also crucial to remember that, in mathematics, the order of operations must be strictly followed to ensure accurate results.
Definition of Like Bases and Unalike Bases
In the study of exponents, the terms like bases and unalike bases often come up. Like bases refer to expressions with the same base number, such as 2³ and 2⁴. Unalike bases, on the other hand, have different base numbers, such as 2³ and 3⁴.
Difference Between Adding Exponents with Like Bases and Unalike Bases
When adding exponents with like bases, the base remains the same while the exponents are added. However, when adding exponents with unalike bases, the exponents are not added; instead, each base is raised to its exponent, and the results are added together.
Steps to Add Exponents
Adding exponents involves a series of straightforward steps. Start by identifying whether the bases are the same. If they are, add the exponents while keeping the base the same. If the bases are different, raise each base to its exponent and add the results.
Steps for Adding Exponents with Like Bases
- Identify that the bases are the same.
- Keep the base number the same.
- Add the exponents.
Steps for Adding Exponents with Unalike Bases
- Identify that the bases are different.
- Raise each base to its respective exponent.
- Add the results.
Examples of Adding Exponents
Let’s look at the process of adding exponents with practical examples.
Example of Adding Exponents with Like Bases
For instance, let’s consider the expression 3³ + 3². Here, the bases are the same (3), so we add the exponents, right? Wrong. We solve each exponent separately, then add the results: (3³ = 27) and (3² = 9). So, 27 + 9 = 36.
Example of Adding Exponents with Unalike Bases
Consider the expression 2³ + 3². Here, the bases are different (2 and 3). So, we calculate each part separately: (2³ = 8) and (3² = 9). Now, we add the results: 8 + 9 = 17.
Practice Problems on Adding Exponents
Test your understanding of adding exponents with these practice problems:
- 4³ + 4²
- 2⁴ + 3³
- 5² + 5³
- 6⁴ + 2²
Conclusion
We trust that this comprehensive guide on adding exponents has been enlightening and has brought some clarity to a topic that often seems complex. At Brighterly, our mission is to make learning mathematics an engaging and enjoyable journey for all learners. We believe that by understanding the core concepts such as exponents, anyone can build a strong mathematical foundation that will serve them well in more advanced studies and real-world problem-solving situations.
Remember, practice is crucial when it comes to mastering mathematics. So, use the examples and practice problems provided to hone your skills. If you encounter any challenges or have more questions, don’t hesitate to reach out to our team of experienced educators. Let’s continue to grow and learn together with Brighterly!
Frequently Asked Questions on Adding Exponents
What does adding exponents mean?
Adding exponents doesn’t involve adding the exponents themselves. Rather, it involves adding the results of base numbers raised to their respective exponents. For instance, when adding 2³ + 2⁴, we don’t add 3 and 4. Instead, we calculate 2³ = 8 and 2⁴ = 16, then add the results: 8 + 16 = 24.
What is the rule for adding exponents?
The rule for adding exponents states that when the base numbers are the same, you simply keep the base and add the exponents. However, it’s important to note that this rule applies when multiplying powers with the same base, not when adding them.
What happens if the bases are different when adding exponents?
When adding exponents with different bases, you cannot add the exponents directly. Instead, each base must be raised to its exponent and the results added together.
What is the zero exponent rule?
The zero exponent rule states that any non-zero number raised to the power of zero equals one. For example, 5⁰ = 1 and 123⁰ = 1. This rule is fundamental in exponent arithmetic.
Information Sources
Online Winter Math Camp for Grades 1-8
Join us to help your kid achieve their full math potential.
Choose kid's grade
Online Winter Math Camp for Grades 1-8
Join our Math Winter Camp and boost your child's math skills with our best tutors.
