• Math tutors
• /
• Knowledge Base
• /
• Center of Circle – Definition, Formula, Examples

Center of Circle – Definition, Formula, Examples

Jo-ann Caballes

Updated on January 1, 2024

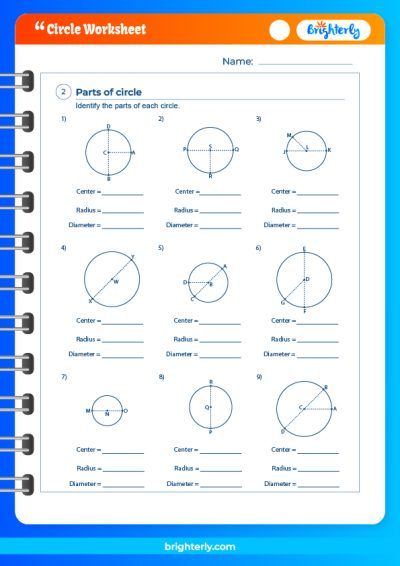
What Is the Center of a Circle?

The center of a circle is like the heart of a circle – it’s the exact middle point from which every edge of the circle is the same distance away. Imagine a spider sitting at the center of its web; no matter which direction the spider moves, it’s always the same distance from the outermost circle of the web.

Circles Geometry Worksheet

Circles Worksheets

Center of a Circle: Formula

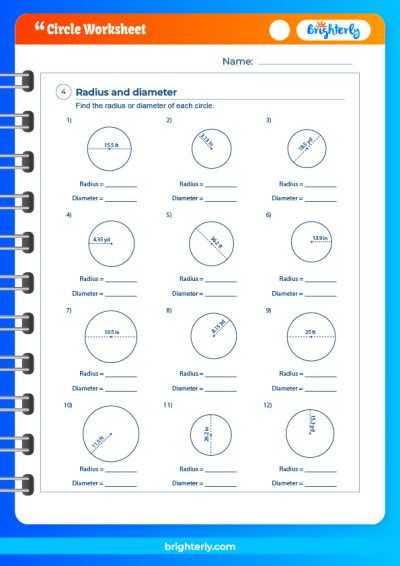
To find the center of a circle, you don’t always need a special formula. The center is simply the point that is equally far from every edge of the circle. If you know the radius—the distance from the center to any point on the edge—you can describe any circle. The general formula for a circle’s radius when you know the diameter (the widest distance across the circle) is this: radius = diameter / 2. This works because the radius is always half the length of the diameter.

Equation of a Circle with Center at Origin

Now, if you place the center of the circle at the very beginning of the number line, which mathematicians call the origin (0,0), the circle’s equation looks like this: x² + y² = r². This is like saying, “If we take any point on the circle and measure how far it is from the center horizontally (x) and vertically (y), and we square those numbers and add them together, it will equal the square of the playground’s radius.”

How to Find the Center of a Circle

How to Find the Center of Circle with Two Points?

Let’s say you have two swings on the playground (two points on the circle’s edge) and you want to find where to place the roundabout (the circle’s center). You would draw a line connecting the two swings and then draw another line right in the middle of that one, going in the opposite direction. Where this second line crosses the first line is where you should place the roundabout!

Practice Questions on Center of a Circle

1. If the diameter of a circle is 10 units, what is the radius?
2. Write the equation of a circle with a radius of 5 units and the center at the origin.
3. How can you find the center of a circle if you know the diameter?

Frequently Asked Questions on Center of a Circle

What is the center of a circle called?

The center of a circle is just called the “center.”

Can a circle have more than one center?

No, every circle has only one center.

Is the center of a circle always at the point (0,0)?

No, the center can be at any location, but it’s at (0,0) when it’s at the origin of a graph.

Jo-ann Caballes

12 articles

As a seasoned educator with a Bachelor’s in Secondary Education and over three years of experience, I specialize in making mathematics accessible to students of all backgrounds through Brighterly. My expertise extends beyond teaching; I blog about innovative educational strategies and have a keen interest in child psychology and curriculum development. My approach is shaped by a belief in practical, real-life application of math, making learning both impactful and enjoyable.