Division – Definition, Properties, and Examples
reviewed by Jo-ann Caballes
Updated on November 15, 2024
The division is one of the basic math actions that allow students to equally divide anything: from slices of pizza for a company to determining the average amount of stars in the nearest galaxies.
Use this article to determine division meaning in math and how to explain it to the child.
What Is Division?
Division is a mathematical operation involving splitting a quantity into multiple equal parts. It is often represented by the symbol ÷. This action is a counterpoint to multiplication.
The Definition of Division
Division definition is simple since it’s a mathematical operation that involves the sharing of an amount into equal-sized groups. It’s a versatile action that can be applied to any number except 0.
The main division rule says that “no one can divide by 0, as the result will be 0”. Keep that in mind while solving problems.
How Does Division Work?
As we said, division works by breaking a quantity into multiple equal parts. If you want to divide 12 by 4, the result will be 3. There is no “Johny gets 4 slices of pizza, Michael receives only 2, while Miranda gets 6 slices”, as this operation is not true division. True math division splits everything equally.
Division: Mathematical Notation
There are multiple ways how math division can be represented mathematically:
1. The division symbol (÷) is the most common way.
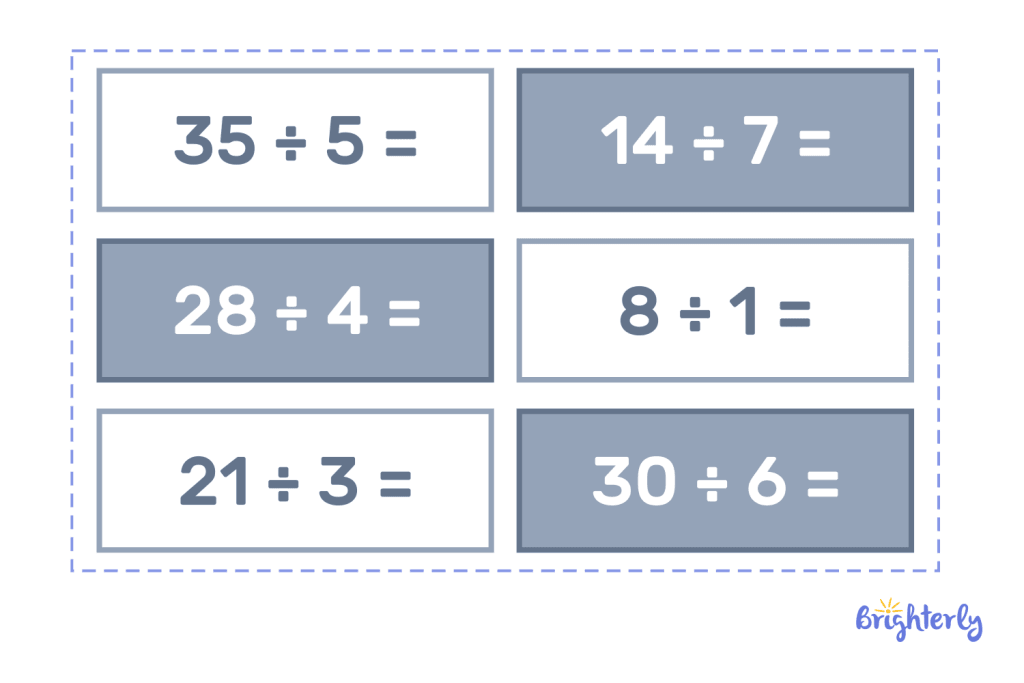
2. Fraction notation, where the dividend is the numerator, and the divisor is the denominator.
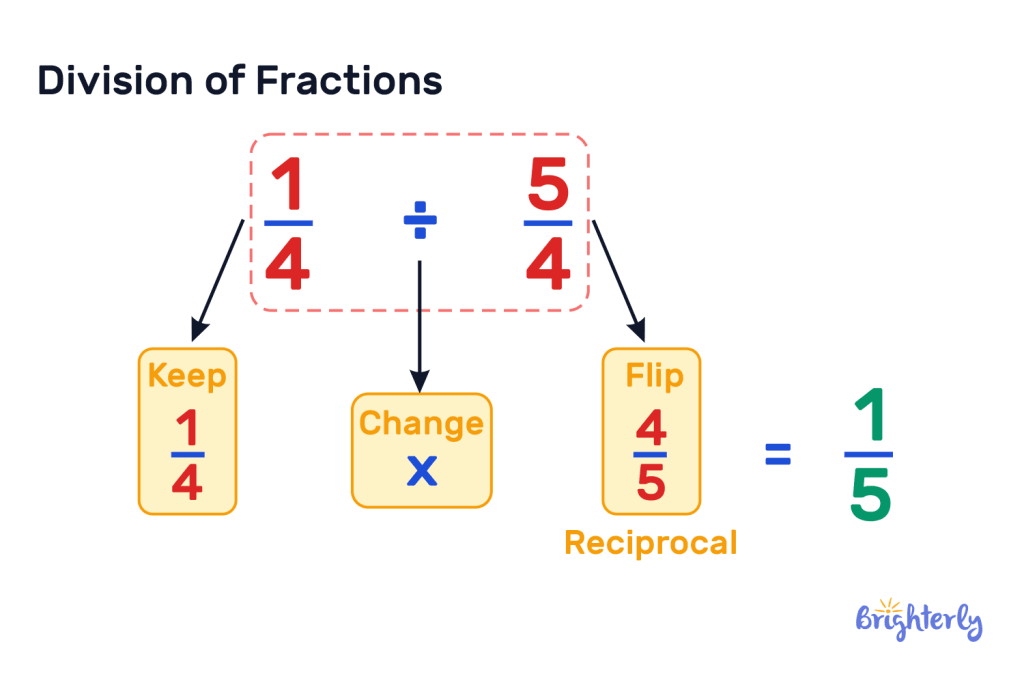
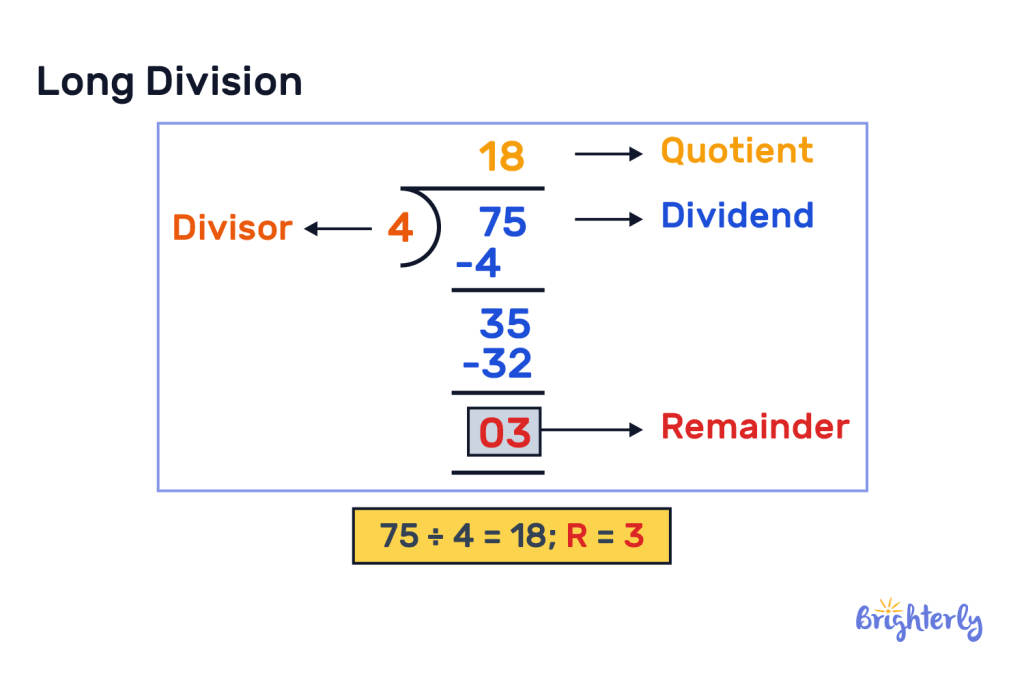
3. The Long Division method is used to divide large numbers with a series of steps to find the quotient.
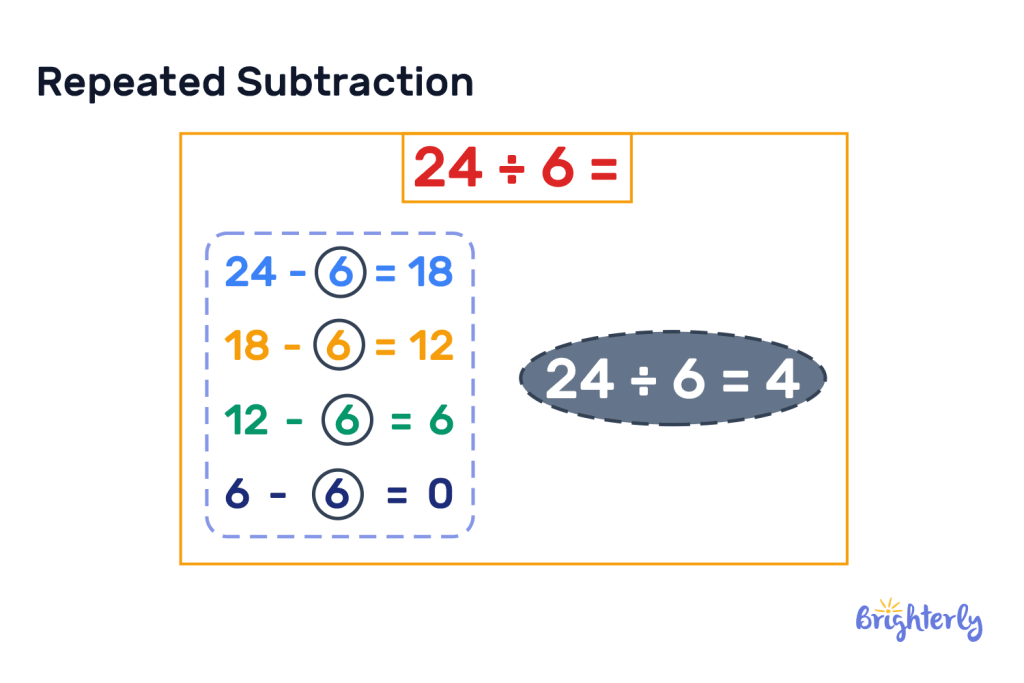
4. Repeated subtraction, where division can be shown as repeated subtraction. You repeatedly subtract the divisor from the dividend until you reach 0. For example, to divide 12 by 3, you can subtract 3 from 12 four times: 12 – 3 – 3 – 3 – 3 = 0.
Real-life Examples of Division
There are various division examples in real life. We’ve shared the most common ones so it is easier for your child to understand the parts of the division equation and the logic behind it.
- Sharing a pizza among a company. Imagine that you need to share 8 slices between 4 friends. You may represent it as 8 ÷ 4 =2.
- Determine the average. If a student gets 80,90, and 100 during three tests, you can average by sum and divide those results. It will result in an equation (80+90+100)÷3=90. Decoding parts of the division equation shows us that a student gets 90 degrees on average.
Names for Numbers in Division Equation
- Dividend: This is the number being divided.
- Divisor: This is the number that divides the dividend.
- Quotient: This is the result of the division.
Division Formula
The division formula has 3 main components: dividend, divisor, and quotient. The whole process can be represented as:
Dividend ÷ Divisor = Quotient
If you have 12 cookies and want to share them equally among 3 friends, you would divide 12 by 3. In this division equation, 12 is a dividend, 3 is a divisor, and 4 is a quotient.
Properties of Division
- Identity Property of Division
- Zero Property of Division
- Commutative Property of Division
- Associative Property of Division
Identity Property of Division
The identity property of division claims that if you divide any number (except 0) by 1, the result is the original number. It’s one of those division rules that is easy to understand since if you have 10 apples and only one person in line, that person gets all 10 apples.
Zero Property of Division
The zero property of division claims that if you divide 0 by any number (except 0), the result is 0. If there are 10 apples and no person in the line, there is no one to get them, so the result is 0.
Dividing a number by 0 is undefined. This division example has no meaning, if there are 0 apples and 0 people in line, there is no purpose for calculating it.
Commutative Property of Division:
The division is not commutative, so the order of numbers really matters. If you want to divide 10 apples between 2 people, they will get 5 fruits. But if you decide to divide 2 apples between 10 people, each of them gets only 0,2 (20%) of the apple.
This property shows that the placement of division equation parts is crucial since 10 ÷ 2 ≠ 2 ÷ 10
Associative Property of Division
The associative property of division claims that this action is not associative. that the grouping of numbers in a division problem matters. For example, (12 ÷ 4) ÷ 2 ≠ 12 ÷ (4 ÷ 2). In the first case, you’ll get 1,5, while in the second situation, the result is 6.
Solved Math Tasks: Examples
Solved math problem 1
You have 15 balloons and need to divide them between 5 kids. How many balloons does each kid get?
Answer:
Let’s recap the names of the numbers in the division. The number of balloons is the dividend. The number of kids is the divisor. The number of balloons for each kid is the quotient.
So, the overall formula will look like:
15÷5=3
|
Therefore, each kid gets 3 balloons. |
Solved math problem 2
You have 18 gray pencils and need to divide them between 6 kids in the group.
Answer:
The division meaning in math is to share something equally. So it can’t be a situation where someone gets 10 pencils while others need to share the remaining 8.
So, the answer will look like
18÷6=3
|
It means that each kid gets 3 pencils. |
Solved math problem 3
You have 160 stickers and 2 school classes with 20 people in each. How many stickers each kid will get?
Answer:
We define division as the equal sharing of something between the divisors. To get the answer, we need to determine the overall number of kids in both classes, which will be 20 x 2 =40.
To find the answer to the original question, we need to divide 160 by 40.
160÷40=4
|
So, each kid will get 4 stickers. |
Division: Practice Math Problems
Division: Worksheets
Now that you know parts of division and how it works, it’s time to practice with division worksheets. They don’t look like traditional school math problems but rather than games. They are free to download, so you can print them to ensure that your child understands crucial division steps and other aspects of this math action.
- Division facts worksheets
- Multiplication and division worksheets grade 3
- Multiplication and division fact families worksheets
- 6th grade division worksheets
Struggling with Division?
- Does your child struggle to understand the concept of division?
- Try learning with an online tutor.
Choose kid's grade
Is your child having trouble grasping division basics? An online tutor could provide the necessary assistance.
