• Math tutors
• /
• Knowledge Base
• /
• Exponential Form – Meaning, Definition With Examples

Exponential Form – Meaning, Definition With Examples

Welcome to Brighterly’s fun journey into the world of exponential form. It’s a math trick for making sense of really big or tiny numbers. We’re going to see how a base and an exponent can simplify numbers and make them easier to work with. We’ll also look at how to switch between exponential form and logarithmic form. This guide is perfect for young math enthusiasts or anyone looking to brush up on their number skills.

What Is Exponential Form?

Exponential form is a way of writing numbers using a base and an exponent. The base is the main number, and the exponent, written as a superscript, tells how many times to multiply the base by itself. For example, 2³ (read as “two to the power of three”) means 2 multiplied by itself two more times, resulting in 8. This form is useful for expressing very large or small numbers compactly.

For negative exponents, like 10^(−2), the expression means 1 divided by the base raised to the positive exponent, which in this case is 1/10^(-2) or 0.01. Exponential form simplifies calculations and understanding in science and mathematics. It’s a key concept in various mathematical applications, from simple calculations to complex scientific equations.

This method helps students grasp the magnitude of numbers, making it easier to handle large values like distances in space or small ones like microscopic measurements.

Basic Concept of Exponential Form

Exponential form is a way of writing numbers that are too large or too small to be conveniently written in standard form. It’s expressed as a number multiplied by 10 raised to an exponent. For example, the number 300 can be written in exponential form as 3 x 10². This method simplifies calculations and understanding of large or small values.

Writing Numbers in Exponential Form

To write a number in exponential form, you identify the base number and the exponent. The base number is what is being multiplied, and the exponent tells how many times to multiply the base number by itself. For instance, 10⁴ means 10 is multiplied by itself four times (10 x 10 x 10 x 10).

Converting Exponential Form to Logarithmic Form

Converting exponential form to logarithmic form involves rewriting the expression so that the exponent becomes the subject of the equation. For example, if you have 2³ = 8, in logarithmic form it becomes log₂8 = 3. This shows that 3 is the power to which 2 must be raised to produce 8.

Converting Logarithmic to Exponential Form

The process of converting logarithmic form to exponential form is the reverse of the above. For example, from log₂8 = 3, we can convert it back to 2³ = 8. This is helpful in solving logarithmic equations.

Exponential Form to Radical Form

Exponential form can also be converted to radical form, which is another way to represent exponents. For instance, 2³ can be written as the cube root of 8, or √³8. This is useful in simplifying complex algebraic expressions.

Standard Exponential Form

Standard exponential form is typically used in scientific notation. It involves writing numbers as a product of a number between 1 and 10 and a power of 10. For example, 0.00045 can be written in standard exponential form as 4.5 x 10⁻⁴.

Characteristics and Examples

Exponential form is characterized by two main components: a base and an exponent. Here are some key characteristics and examples to illustrate this concept:

1. Base and Exponent: In an expression like $aⁿ$, ‘a’ is the base and ‘n’ is the exponent. The base can be any real number, and the exponent indicates how many times the base is multiplied by itself.

   • Example: In 3⁴, 3 is the base, and 4 is the exponent. It means $=81$.

2. Negative Exponents: A negative exponent indicates that the base is divided instead of multiplied. It’s equivalent to the reciprocal of the base raised to the opposite positive exponent.

   • Example: 2^(-3) means 1/2³=​1/8​.

3. Fractional Exponents: These exponents represent roots. For example, an exponent of 1/2 is equivalent to the square root.

   • Example: 9^(/1)​ is the same as √9​=3.

4. Zero as an Exponent: Any non-zero number raised to the power of zero equals 1.

   • Example: $=1$.

5. Exponent of 1: Any number raised to the power of 1 is the number itself.

   • Example: $=7$.

These characteristics highlight the versatility of exponential form in mathematics. It simplifies the representation and calculation of complex numbers, making it a fundamental concept in both basic and advanced mathematics.

Exponential Form Examples

1. 5² = 25 (5 multiplied by itself once)
2. 10⁻³ = 0.001 (1 divided by 10, three times)

Exponential Form Table

Below is a table that illustrates different numbers in exponential forms. This table is a handy reference for understanding how exponential notation is applied to various numbers:

Number in Standard Form	Exponential Form
100	10²
1,000	10³
10,000	10⁴
0.01	10⁻²
0.001	10⁻³
0.0001	10⁻⁴
8	2³
27	3³
1/8	2⁻³
1/27	3⁻³

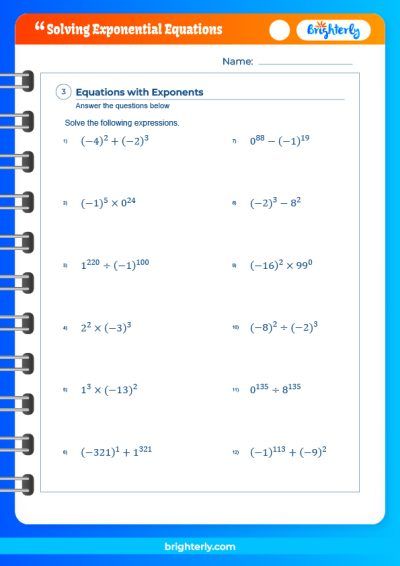
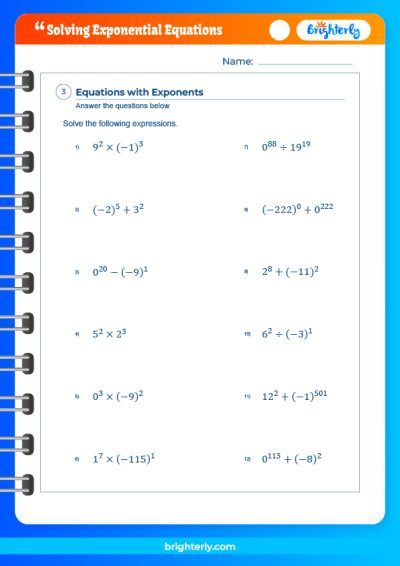
Practice Questions on Exponential Form

1. Convert 1000 to exponential form.
2. Rewrite 3.6 x 10⁴ in standard form.

FAQs on Exponential Form

What is an exponent?

An exponent in an exponential form indicates how many times the base number is multiplied by itself.

Why is exponential form useful? 

It simplifies the representation and calculation of very large or very small numbers.