Volume of a Rectangular Prism – Definition with Examples
Updated on January 9, 2024
Welcome to another fascinating journey with Brighterly, where we bring the wonder of mathematics alive for children! In this blog post, we are going to explore the concept of the volume of a rectangular prism. This concept is a fundamental part of geometry, and understanding it opens up the world of three-dimensional shapes to young learners.
As part of our mission here at Brighterly, we strive to simplify complex mathematical concepts and make them accessible and enjoyable for kids. We believe in learning through practical examples and interactive exercises. That’s why, in this post, we’ll also be providing real-life examples and practice questions to help kids understand and apply this concept better.
So, sit back, and let’s delve into the world of rectangular prisms and their volumes! Remember, with Brighterly, learning math is not just easy – it’s fun too!
What Is a Rectangular Prism?
Let’s delve into the fascinating world of 3D geometry, starting with one of its basic structures – the Rectangular Prism. A rectangular prism is a three-dimensional shape. Picture a box or a book; both are perfect examples of a rectangular prism in the real world. It has six faces, and all of these faces are rectangles. The edges are all perpendicular to each other, and it possesses both length, breadth, and height, which sets it apart from a two-dimensional figure.
The defining factor that makes a prism a rectangular prism is that the bases are rectangles. If you were to slice the prism parallel to its base, the cross-section you’d be left with would also be a rectangle. The properties of a rectangular prism make it an interesting object to study, especially when we start discussing its volume. But before we dive into that, let’s understand what we mean by the term ‘volume’.
Volume of Rectangular Prism Formula
The volume of an object in 3D space is essentially the amount of space it occupies. In the context of a rectangular prism, it’s like asking, “How many unit cubes can fit inside this box?” The unit could be anything from a cubic meter to a cubic millimeter, depending on the context.
Now, to calculate the volume of a rectangular prism, we need a formula. The beauty of math is that once you understand the concept, the formula becomes intuitive. So, how do we derive the formula for the volume of a rectangular prism?
The Formula for Volume of a Rectangular Prism
In the case of a rectangular prism, the volume can be calculated by simply multiplying its length, width, and height. It’s equivalent to arranging unit cubes in the prism, covering its length, breadth, and height entirely. This simple yet effective formula stands as:
Volume = Length × Width × Height
It’s important to note that all three dimensions need to be in the same unit to ensure the accuracy of the calculation. The units for the volume are always cubic units because we are considering three dimensions.
How to Find the Volume of a Rectangular Prism?
Finding the volume of a rectangular prism is a three-step process.
- Measure the length, width, and height of the prism. Make sure all measurements are in the same unit.
- Plug the measurements into the formula: Volume = Length × Width × Height.
- Compute the multiplication to get the volume.
It’s as straightforward as that! Now let’s try to understand this with a few examples.
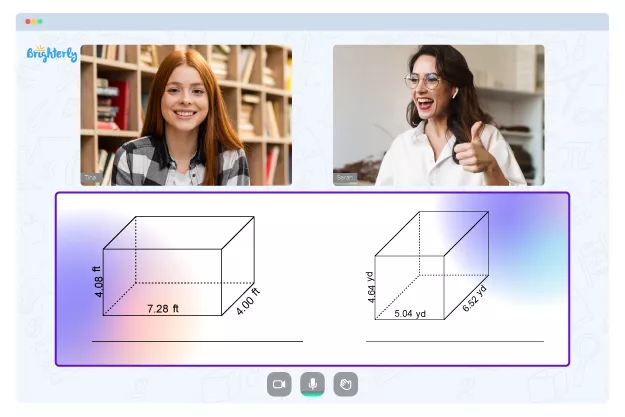
Volume of Rectangular Prism Examples
To illustrate, let’s consider a rectangular prism with a length of 4 units, a width of 3 units, and a height of 2 units. By substituting these values into our formula, we get:
Volume = 4 units × 3 units × 2 units = 24 cubic units
The volume of this rectangular prism is therefore 24 cubic units.
Let’s take another example: a rectangular prism with dimensions 5 units by 6 units by 7 units.
Volume = 5 units × 6 units × 7 units = 210 cubic units
This prism has a volume of 210 cubic units.
Practice Questions on Volume of a Rectangular Prism
For further practice, try calculating the volume of rectangular prisms with the following dimensions:
- Length = 6 units, Width = 4 units, Height = 2 units
- Length = 8 units, Width = 3 units, Height = 7 units
- Length = 5 units, Width = 5 units, Height = 5 units
Conclusion
Learning about the volume of a rectangular prism, we venture into the awe-inspiring world of three-dimensional geometry, a significant domain in mathematics with wide-ranging real-world applications. Recognizing a rectangular prism, understanding its properties, and knowing how to calculate its volume can help children relate their learnings to everyday scenarios. This could be as simple as figuring out how much a gift box can hold or as practical as determining the capacity of a storage unit.
At Brighterly, we believe that fostering such an understanding is a crucial step in children’s educational journey. We make it our mission to present these mathematical concepts in an engaging and accessible manner, bridging the gap between abstract math and tangible reality.
In our journey through this article, we’ve tackled the definition of a rectangular prism, discussed its volume, and have broken down the volume calculation process into simple, understandable steps. We’ve also looked at practical examples and provided practice questions to strengthen this newfound knowledge.
Remember, at Brighterly, we’re not just about learning – we’re about experiencing the joy of understanding, and we sincerely hope this blog post has contributed to that joy.
Frequently Asked Questions on Volume of a Rectangular Prism
What is the formula for the volume of a rectangular prism?
The formula for the volume of a rectangular prism is as straightforward as the shape itself. It’s Volume = Length × Width × Height. Each dimension corresponds to one of the three dimensions of the rectangular prism, and multiplying them together gives us the amount of space the prism occupies.
Can the volume of a rectangular prism be negative?
The volume of a rectangular prism can never be negative. It represents the amount of space taken up by the prism in three-dimensional space. Just like you can’t have a negative amount of space, the volume, too, is always a positive value.
Do the length, width, and height have to be in the same unit when calculating the volume of a rectangular prism?
Yes, the measurements of length, width, and height should indeed be in the same unit when calculating the volume of a rectangular prism. This is because volume is a three-dimensional measure, and mixing units can lead to inaccurate results. So, whether you’re working with inches, feet, or meters, make sure all dimensions are consistent.
Information Source:
Troubles with Numbers?
- Does your child struggle to grasp numbers lessons?
- Try studying with an online tutor.
Choose kid's grade
Is your child having difficulties with understanding numbers? An online tutor could provide the necessary guidance.
