Least common multiple: Definition and Practice Problems
reviewed by Jessica Kaminski
Updated on October 4, 2024
We get multiples by multiplying integers with other whole numbers like 1,2,3, etc.
Common multiples are the same numbers that appear within the multiples of two or more integers.
Let’s explore the meaning of LCM in math, methods to find it, examples, and more.
What is the least common multiple?
The least common multiple (LCM) is the smallest number that appears within the multiples of two or more numbers.
For example, multiples of 2 are 2, 4, 6, 8, 10, 12, 14, 16, 18, 20….
Multiples of 5 are 5, 10, 15, 20, 25, 30…..
This means the common multiples of 2 and 5 are 10, 20, and so on.
Therefore, 10 is the least common multiple of 2 and 5
This is because it is the lowest multiple that occurs in the lists of both integers.
Least common multiples are most helpful for finding the common denominators whenever we want to do addition or subtraction between two or more fractions.
How to find the least common multiple (LCM)?
Now that we’ve covered the definition of least common multiple, below are the methods for finding it:
Listing the Multiples
List the multiples of each integer, identify their common numbers, and select the smallest as the LCM.
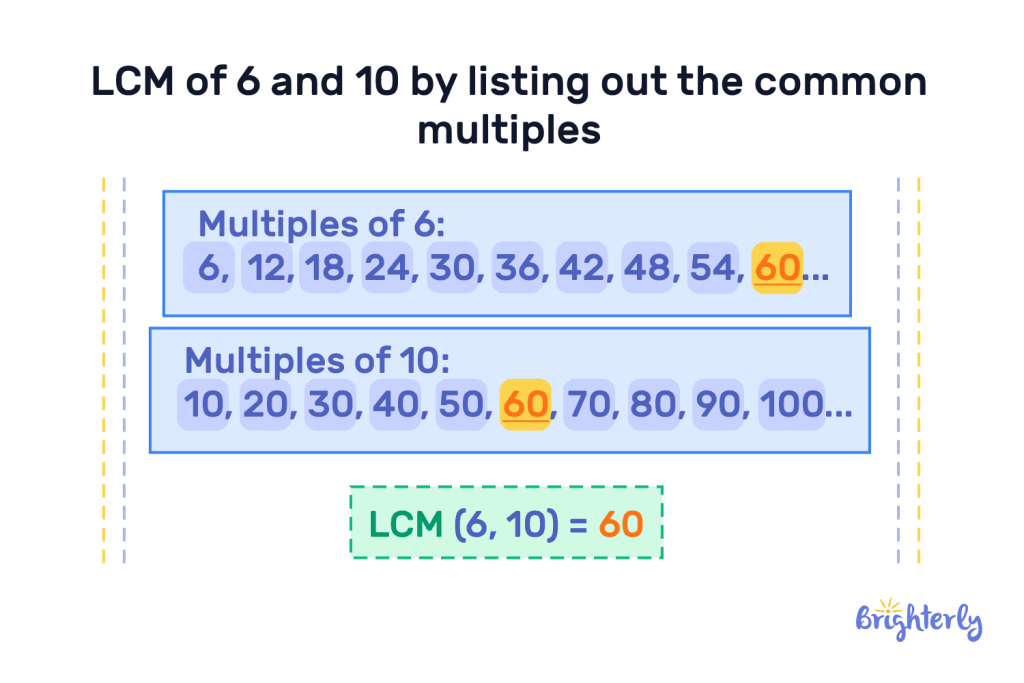
This image shows that 30 is the least common multiple for 6 and 10.
Prime Factorization
Prime numbers can only be divided by 1 and itself. Prime factors are prime numbers that, when multiplied, can make an integer.
For this method;
- List the prime factors that make up the given numbers.
- Identify the common and unique factors.
- Multiply the common and unique factors to get the least common multiple.
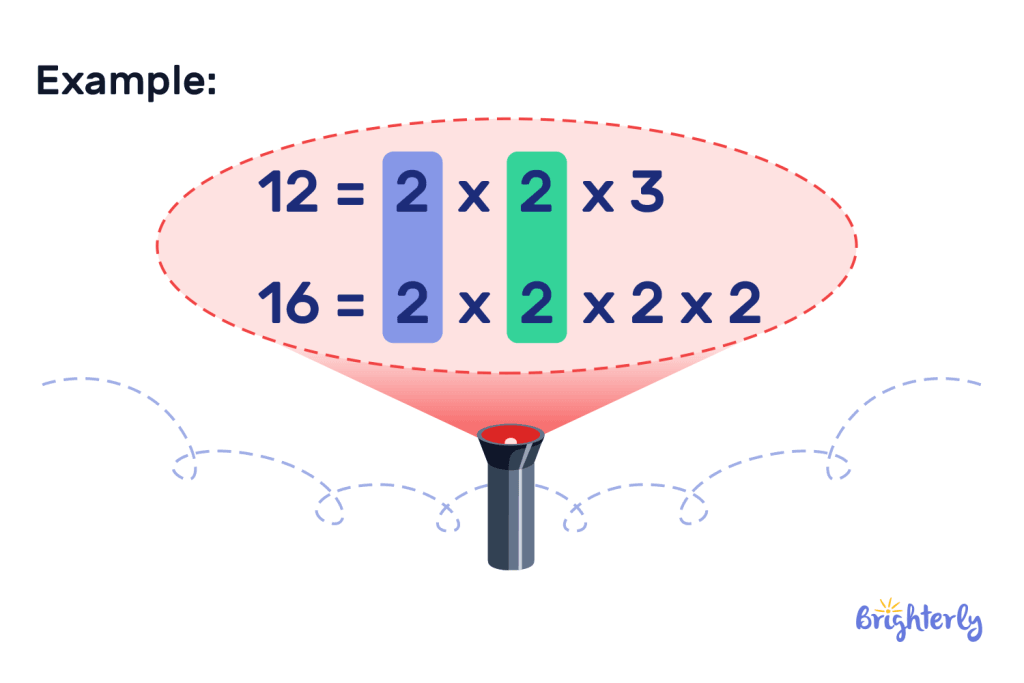
To get the LCM, we multiply the common factors (2 × 2) by the unique factors (2 × 2× 3)
LCM (12,16) = 48
Division
Divide the numbers by their common factors until we get to 1, i.e., they are no longer divisible. Next, multiply the factors and the leftover numbers (if applicable) to get the LCM.
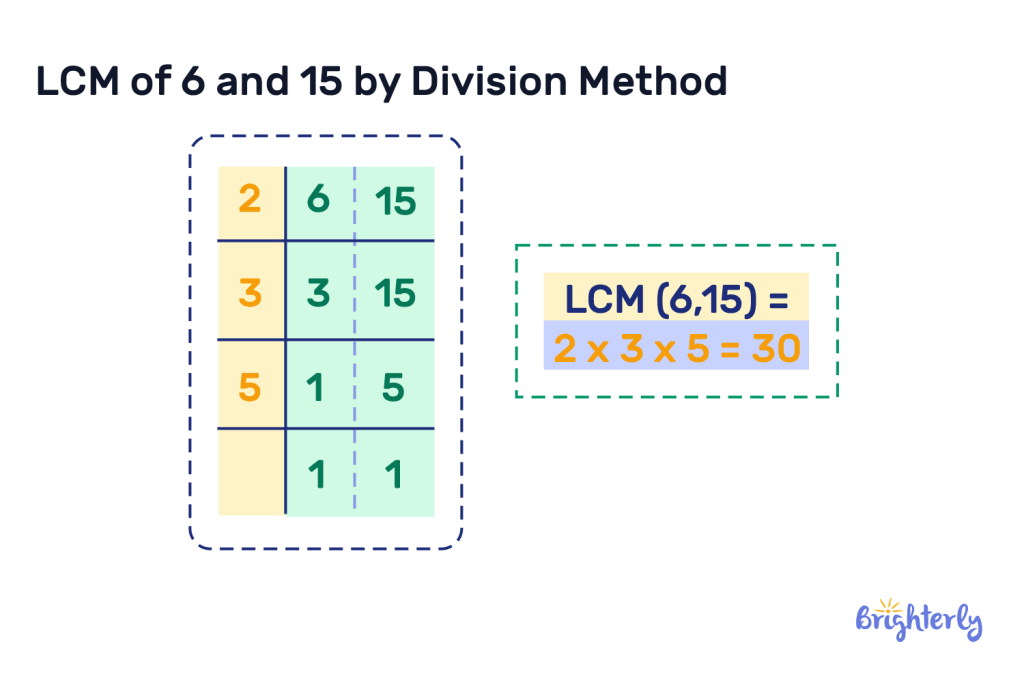
When the numbers have been divided into their lowest common factor, we multiply the factors to get the LCM.
Relationship between LCM and HCF:
The LCM is the smallest multiple that occurs across two or more numbers and the highest common factor (HCF) is the greatest number that can divide two or more numbers.
To determine their relationship, let’s presume we have been given two numbers: A & B
The formula for determining the relationship between the LCM and HCF is:
LCM (a, b) × HCF (a, b) = a × b
How to do the least common multiple: Formula
This formula finds the least common multiple without using the above methods.
All that’s needed are the numbers and their highest common factor (HCF).
Taking a & b as our given numbers, here is the LCM formula:
LCM = (a x b) ÷ HCF(a,b)
To get the LCM, we multiply the given numbers and divide them by the HCF of the numbers.
Solved Math Tasks: Examples
Below are some LCM examples using the methods above:
Solved math problem 1
Find the LCM of 12 and 21 employing the Prime Factorization method.
Answer
12 = 2 × 2 × 3
21 = 3 × 7
LCM (12, 21) = 2 × 2 × 3 × 7
| LCM( 12, 21) = 84 |
Solved math problem 2
Find the LCM of 10, 15, and 20 with the Division method
Answer
| 2 | 10 | 15 | 20 |
| 2 | 5 | 15 | 10 |
| 5 | 5 | 15 | 5 |
| 3 | 1 | 3 | 1 |
| 1 | 1 | 1 |
LCM = 2× 2 × 5 × 3 = 60
| LCM (10, 15, 20) = 60 |
Solved math problem 3
Find the least common multiple for the numbers 3 and 6 when the HCF is 3
Answer
LCM (3,6)= (3× 6) ÷ 3
LCM (3,6) = 18 ÷ 3
LCM (3,6)= 6.
| Thus, 6 is the LCM of 3 and 6. |
Least common multiple: Practice Math Problems
Least common multiple: worksheets
Brighterly offers worksheets that cover LCiM in math as well as other topics in Factors and Multiples:
Online Winter Math Camp for Grades 1-8
Join us to help your kid achieve their full math potential.
Choose kid's grade
Online Winter Math Camp for Grades 1-8
Join our Math Winter Camp and boost your child's math skills with our best tutors.
