• Math tutors
• /
• Knowledge Base
• /
• Trapezoid – Definition, Types, Properties, Examples, FAQs

Trapezoid – Definition, Types, Properties, Examples, FAQs

Jessica Kaminski

reviewed by Jo-ann Caballes

Updated on October 9, 2024

Looking to improve your math knowledge? Brighterly is here to help!

As part of our knowledge base series on shapes, we’re exploring trapezoids shapes today.

In this article, we’ll share the trapezoid meaning, the properties of trapezoids, different types of trapezoids, solved math problems and trapezoid practice problems.

What is trapezoid?

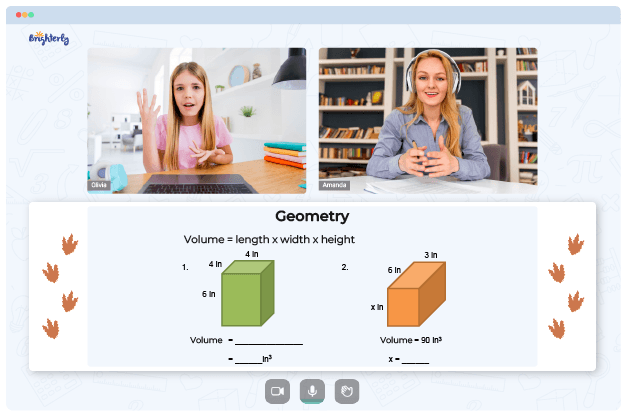
A trapezoid is a type of quadrilateral. The definition of a trapezoid is a 2D closed shape with four sides and one pair of parallel sides, known as the base. The non-parallel sides are known as the legs or laterals. All trapezoid interior angles add up to 360°.

Trapezoid: exclusive & inclusive definition

There are two definitions of a trapezoid, exclusive and inclusive.

Exclusive definition

The exclusive definition of a trapezoid says they have no opposite parallel pairs.

Inclusive definition

The inclusive definition of a trapezoid says it has at least one pair of opposite parallel sides. By this definition, parallelograms can be classed as trapezoids.

This article will be using the inclusive definition of trapezoids shapes.

Types of trapezoid

There are three different types of trapezoids:

• Right trapezoid
• Isosceles trapezoid
• Scalene trapezoid

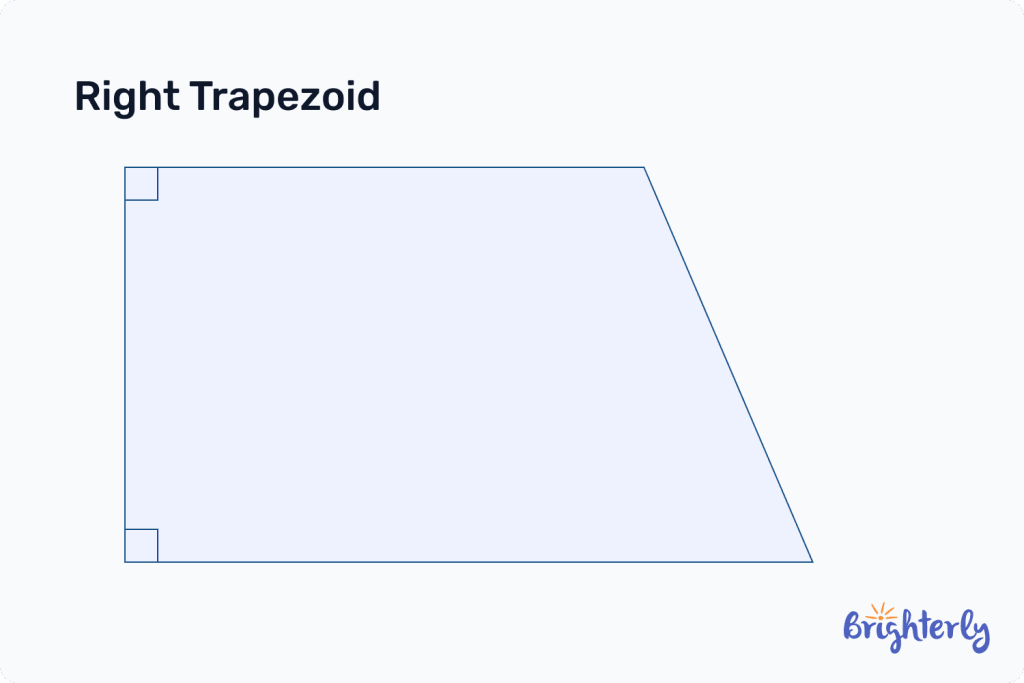
Right trapezoid

A right trapezoid has one leg perpendicular to both its bases. This creates two right angles, one at each of its bases, so it’s also known as a right-angled trapezoid.

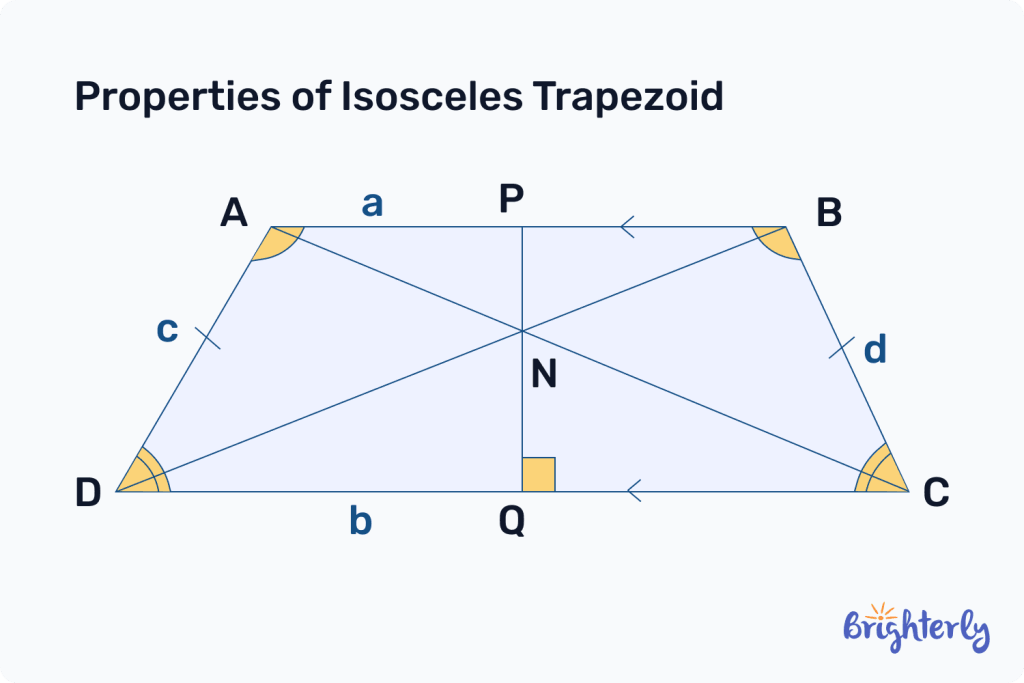
Isosceles trapezoid

Isosceles trapezoids shapes have parallel top and bottom lines (bases). The other two lines are equal length, but aren’t parallel. This means the base angles and diagonals of an isosceles trapezoid are equal.

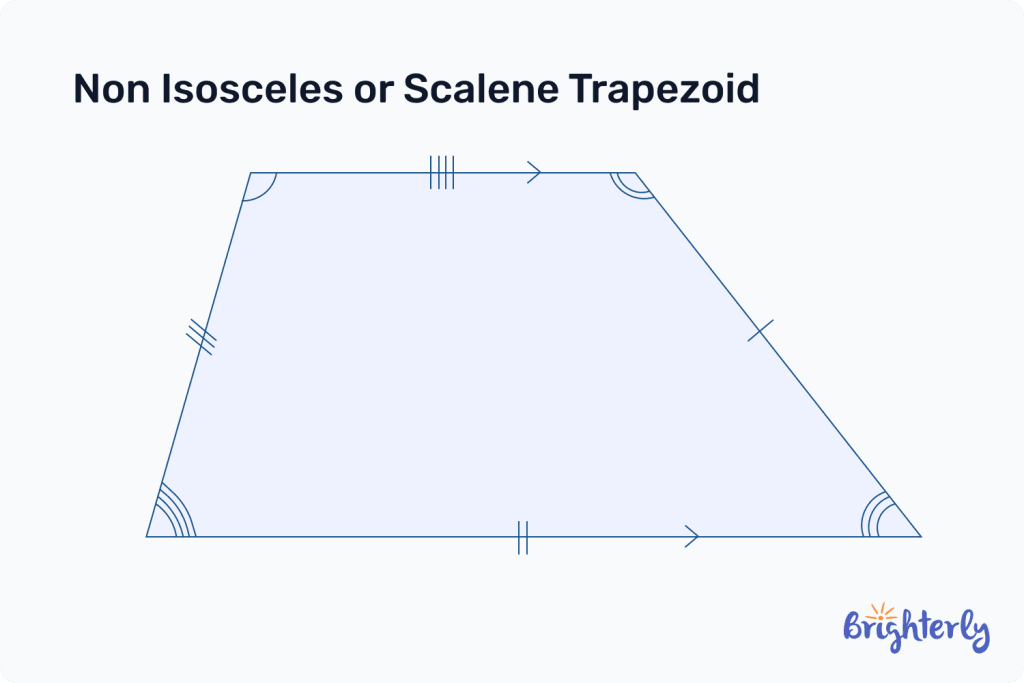
Scalene trapezoid

A scalene trapezoid has sides that are different lengths and non-congruent. This means each of its four angles is different. It also has a pair of parallel sides and a pair of non-parallel sides. It’s also known as a non-isosceles trapezoid.

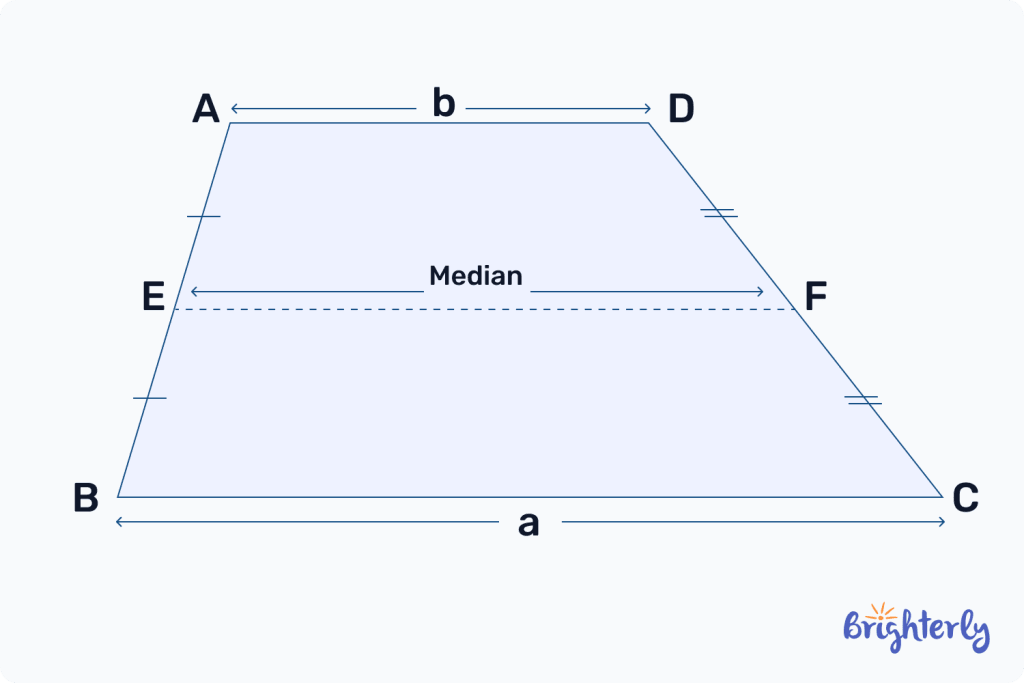
Median of trapezoid

The median of a trapezoid is equal to half the sum of both its bases and parallel to them.

Properties of a trapezoid

The properties of trapezoids make them different from other types of quadrilaterals. These properties are:

• The top and bottom line (bases) of trapezoids shapes are parallel. 
• An isosceles trapezoid has opposite sides of the same length.
• The median of trapezoids is parallel to both its bases.
• If both opposite sides are parallel, a trapezoid can also be a parallelogram.
• A square can also be a trapezoid under the inclusive definition.
• A rectangle can also be a trapezoid under the inclusive definition.
• All trapezoid interior angles add up to 360°, and the angles on the same side add up to 180°.

Math tutors

Improve your child’s math skill today with Brighterly

Let them be at the top of their class.

Book free lesson

Trapezoid formula

There are two trapezoid formulas you can use to calculate the area and perimeter of a trapezoid.

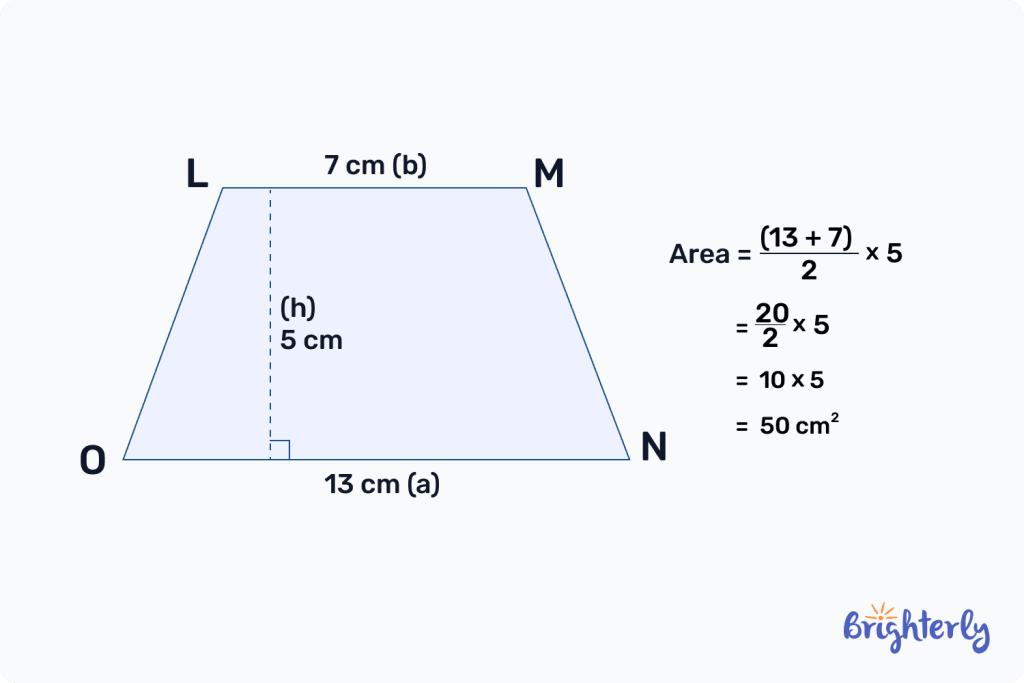
Area of trapezoid

This formula calculates the area of a trapezoid:

Area= ½ (A+B)h

(A = base1, B = base2, h = height).

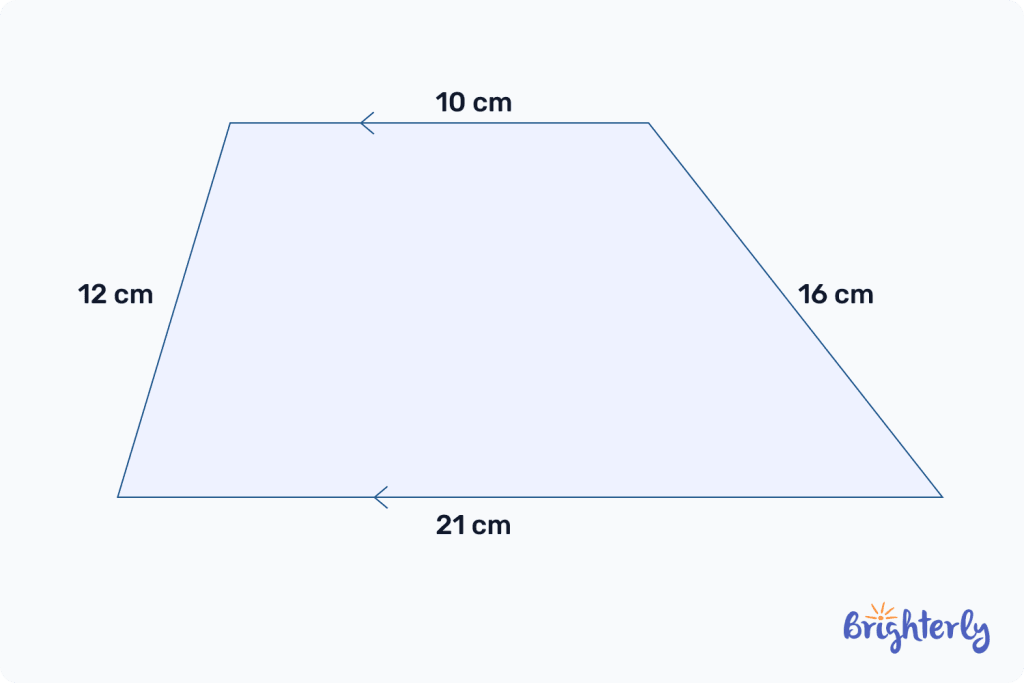
Perimeter of trapezoid

To calculate the perimeter of a trapezoid, simply add all of its side lengths together.

If you don’t know all the side lengths, you can use the Pythagorean theorem to calculate them.

Solved math tasks: examples

Here, we’ve included some solved trapezoid practice problems so you can improve your learning.

Solved math task 1

What is the perimeter of this trapezoid? 

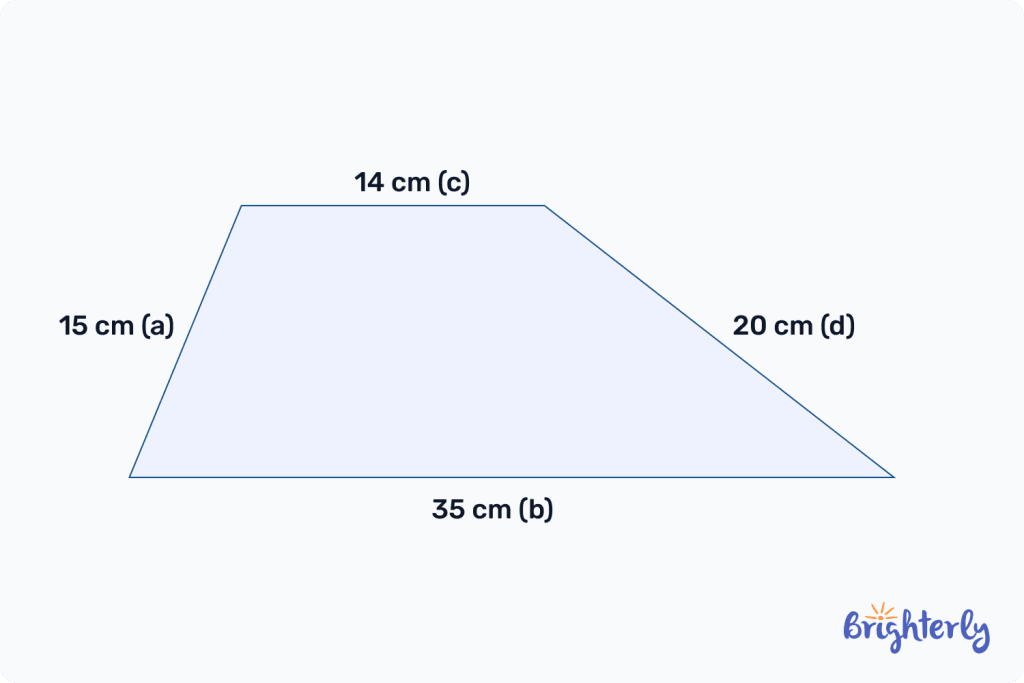
Answer:

Perimeter = a + b + c + d

Perimeter = 15cm + 35cm + 14cm + 20cm = 84cm

Solved math task 2

What is the area of this trapezoid?

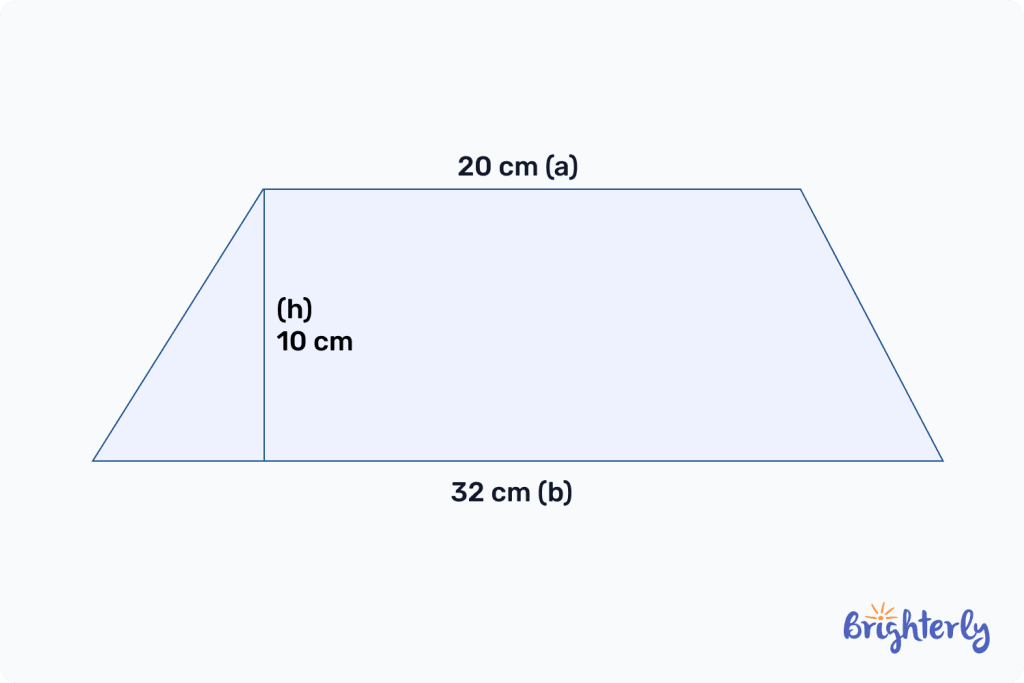
Answer:

Area= ½ of (a+b)h

Area= ½ of (20+32)10    

Area= ½ of 5210

Trapezoid: Practice math problems

Trapezoid worksheets

Brighterly offers a range of math worksheets, including trapezoid problems to solve, to help you put your learning into practice:

• Trapezoid worksheets
• Area of a trapezoid worksheets
• Area and perimeter worksheets
• Identifying shapes worksheets

Jessica Kaminski

72 articles

Jessica is a a seasoned math tutor with over a decade of experience in the field. With a BSc and Master’s degree in Mathematics, she enjoys nurturing math geniuses, regardless of their age, grade, and skills. Apart from tutoring, Jessica blogs at Brighterly. She also has experience in child psychology, homeschooling and curriculum consultation for schools and EdTech websites.